In 1800, Italian physicist Alessandro Volta created the first practical chemical cell, known as the voltaic pile. This groundbreaking invention consisted of alternating layers of zinc and copper discs, separated by cardboard soaked in saltwater. When connected, the voltaic pile produced a steady electric current, demonstrating the conversion of chemical energy into electrical energy. This innovation marked the birth of the first true battery and laid the foundation for future developments in electrochemical cells.
Building on Volta’s pioneering work, British chemist John Frederic Daniell introduced the Daniell cell in 1836. Daniell’s design improved upon the voltaic pile by replacing the saltwater-soaked cardboard with a porous pot containing a copper sulfate solution. The zinc electrode was dipped into dilute sulfuric acid. This configuration provided a more stable electromotive force (EMF) and significantly reduced the polarization effects that plagued earlier designs. The Daniell cell was a major step forward, offering a more reliable and efficient power source.
The mid-19th century saw further advancements in cell technology with the contributions of William Grove and Robert Bunsen. Grove introduced the Grove cell, which used nitric acid and zinc, while Bunsen developed the Bunsen cell, employing sulfuric acid and zinc. Both cells improved the efficiency and reliability of electrochemical power sources, paving the way for more sophisticated applications.
In 1866, French engineer Georges Leclanché invented the Leclanché cell, a design known for its simplicity and practicality. This cell featured a zinc anode immersed in ammonium chloride (NH₄Cl) solution, with a cathode composed of a carbon rod surrounded by manganese dioxide (MnO₂). The Leclanché cell was widely used in telegraphs and doorbells due to its reliability, low cost, and ease of use. Its success demonstrated the potential for electrochemical cells to be integrated into everyday devices.
Around 1887, Carl Gassner introduced the dry cell, a significant advancement over previous designs. Unlike earlier cells that used liquid electrolytes, the dry cell utilized a paste electrolyte, making it more portable and less prone to leakage. The zinc container served as both the anode and casing, while the manganese dioxide and carbon rod formed the cathode. Dry cells became ubiquitous in devices like flashlights, radios, and toys, providing a convenient and reliable source of portable power.
In the 1950s, Lewis Urry developed the alkaline battery, which replaced the acidic electrolyte with an alkaline paste, typically potassium hydroxide. Alkaline batteries offered several advantages over earlier designs, including higher capacity, longer shelf life, and better performance under various conditions. These batteries quickly became the standard for many portable electronic devices, from remote controls to portable radios.
The late 20th century witnessed a revolution in battery technology with the advent of lithium-ion batteries. These batteries use lithium compounds as the anode material and are known for being lightweight and rechargeable. Lithium-ion batteries have become the powerhouse behind modern portable electronics, including smartphones, laptops, and electric vehicles. Their high energy density, long cycle life, and ability to maintain performance over numerous charge-discharge cycles make them indispensable in today’s digital age.
EMF
When you think of electrical circuits, imagine a network of interconnected cells—tiny powerhouses that drive our devices. But what exactly is the Electromotive Force (EMF) of a cell, and how does it impact our circuits?
What Is EMF? EMF is like the cell’s energy passport. It measures how much energy the cell transfers per unit of charge passing through it.
Think of it as the cell’s motivation to push charges around the circuit. Without EMF, our gadgets would stay silent.
Cell Connections: Series and Parallel Imagine you have multiple cells. How do you connect them?
Series connection: Cells line up like a string of pearls. The positive terminal of one cell connects to the negative terminal of the next. In series, EMFs add up. If you connect two 1.5V cells in series, you get a total of 3V. Example: Old-style Christmas lights—they glow in sequence.
Parallel connection: Cells sit side by side, like stars in the night sky. All positive terminals connect, and all negative terminals connect. Each cell gets the full voltage across it. Example: Modern LED fairy lights—they shine independently.
In series, voltage divides among cells. Bulbs may appear dimmer. In parallel, each cell gets full voltage, maintaining brightness.
Series circuits are sensitive. One faulty cell affects the whole chain. Parallel circuits are robust. One cell’s hiccup doesn’t bother the others.
Internal Resistance
When you think of a battery or a cell, you might picture a compact energy source, ready to power your devices. But there’s more to the story—something hidden within the cell itself.
What Is Internal Resistance? Imagine a cell as a tiny power station. It generates an electromotive force (EMF) that pushes charges through a circuit. But wait! Inside the cell, there’s a subtle resistance—a roadblock for those charges. This is the internal resistance. Think of it like friction in a machine—it opposes the flow of current.
Inside the cell, chemical reactions occur. These reactions involve ions moving through the electrolyte. The electrolyte and electrodes (like zinc and copper) have inherent resistance. Even the wires connecting the cell contribute a bit of resistance.
As current flows, some energy is lost overcoming internal resistance. The actual voltage available to the external circuit is less than the cell’s nominal EMF. The energy lost due to internal resistance appears as heat within the cell.
The total voltage across the cell is:
\(\displaystyle\text{Total Voltage} = \text{EMF} – I \cdot r\)
So, the effective EMF is reduced by the internal voltage drop.
Combination of Cells in Series and Parallel
In everyday situations, finding batteries with exactly the voltage you need is not always possible. There are only a limited number of battery types available in the market. So, when you require a specific voltage that isn’t readily available, you can combine two or more batteries in different ways to achieve the desired voltage and current.
There are two fundamental ways to combine batteries: series combination and parallel combination. These two types form the basis for all other combinations.
- Series Combination: When batteries are connected in series, the positive terminal of one battery is linked to the negative terminal of the next. This arrangement adds up the voltages of each battery to produce a higher total voltage. However, the current remains the same across all batteries in the series.
- Parallel Combination: In a parallel combination, the positive terminals of all batteries are connected, and the negative terminals are also connected together. This setup keeps the voltage the same as that of a single battery but increases the total current capacity. It’s like having multiple paths for the current to flow, which collectively can supply more power.
Series Combination
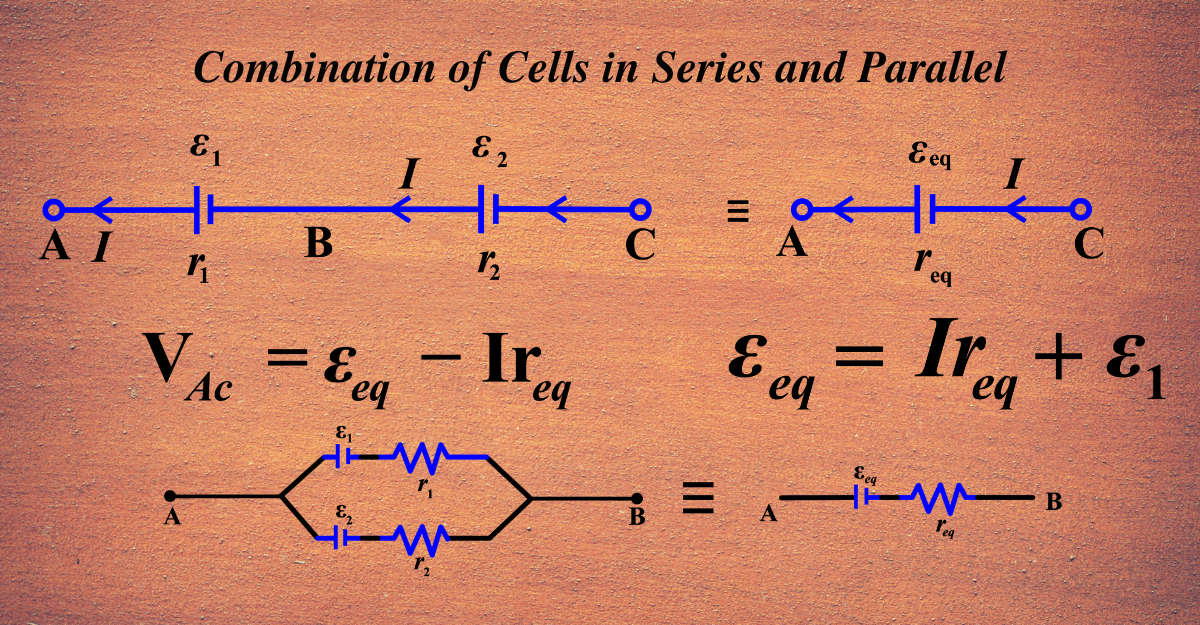
- When cells are connected in series, the positive terminal of one cell is connected to the negative terminal of the next cell, and so on. The same current flows through each cell.
- The total voltage of the series combination is equal to the sum of the voltages of each cell. This is because the potential difference between the terminals of each cell adds up along the circuit.
- The total internal resistance of the series combination is equal to the sum of the internal resistances of each cell. This is because each cell’s internal resistance opposes the current flow along the circuit.
- A single equivalent cell with the same total voltage and internal resistance can replace the series combination of cells. The equivalent cell can provide the same current and power to the external load as the series combination.
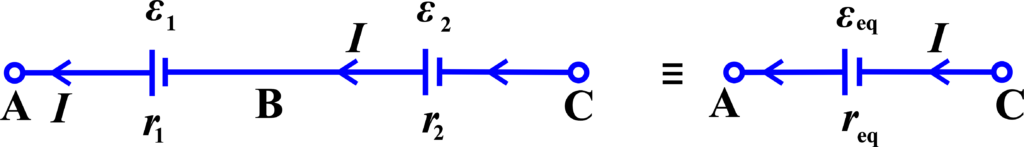
When cells (batteries) are connected in series, the positive terminal of one cell is connected to the negative terminal of the next cell. The overall voltage is the sum of the individual cell voltages, but the current through each cell is the same.
Voltage in Series: The total voltage across cells connected in series is the sum of the voltages of each cell.
Current in Series:
The same current flows through each cell in a series of connections.
Let’s consider (n) cells connected in series. Assume that each cell has an electromotive force (emf) (E) and an internal resistance (r).
For cells in series, the total emf (Etotal) is the sum of the EMFs of each cell.
\(\displaystyle E_{\text{total}} = E_1 + E_2 + \cdots + E_n\)
If each cell has the same emf (E), then:
\(\displaystyle E_{\text{total}} = nE\)
The total internal resistance (Rinternal) is the sum of the internal resistances of each cell.
\(\displaystyle R_{\text{internal}} = r_1 + r_2 + \cdots + r_n\)
If each cell has the same internal resistance (r), then:
\(\displaystyle R_{\text{internal}} = nr\)
When a load with resistance (R) is connected across the series combination of cells, the total voltage (V) across the load is given by:
\(\displaystyle V = E_{\text{total}} – I \cdot R_{\text{internal}}\)
where (I) is the current through the circuit. The current (I) in the circuit can be found using Ohm’s Law. The total resistance in the circuit is the sum of the external resistance (R) and the total internal resistance (Rinternal).
\(\displaystyle I = \frac{E_{\text{total}}}{R + R_{\text{internal}}}\)
Substituting (\(\displaystyle E_{\text{total}} = nE\)) and (\(\displaystyle R_{\text{internal}} = nr\)):
\(\displaystyle I = \frac{nE}{R + nr}\)
The internal resistance of a series combination of cells reduces the circuit’s effective voltage and power output. The advantage of the series combination of cells is that it can increase the voltage and power output of the circuit. The disadvantage of the series combination of cells is that it can increase the internal resistance and energy loss of the circuit.
Parallel Combination
A combination of cells in parallel is a connection of cells where the positive terminals of all the cells are connected, and the negative terminals of all the cells are connected. The same potential difference is applied across each cell in the parallel combination.
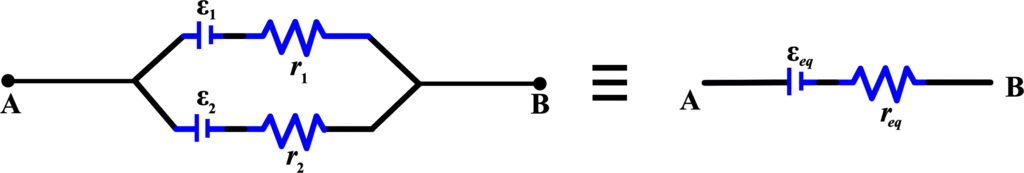
When cells (batteries) are connected in parallel, all the positive terminals are connected together, and all the negative terminals are connected together. The overall voltage remains the same as the voltage of a single cell, but the total current is the sum of the currents from each cell.
Voltage in Parallel: The total voltage across cells connected in parallel is the same as the voltage of each individual cell.
Current in Parallel: The total current is the sum of the currents supplied by each cell.
Let’s consider (n) cells connected in parallel. Assume that each cell has an electromotive force (emf) (E) and an internal resistance (r).
For cells in parallel, the total voltage (Etotal) is equal to the voltage of any single cell, because all cells have the same voltage:
\(\displaystyle E_{\text{total}} = E\)
The total internal resistance (Rinternal) of cells in parallel is found by the formula for resistances in parallel:
\(\displaystyle\frac{1}{R_{\text{internal}}} = \frac{1}{r_1} + \frac{1}{r_2} + \cdots + \frac{1}{r_n}\)
If each cell has the same internal resistance (r), then:
\(\displaystyle\frac{1}{R_{\text{internal}}} = \frac{1}{r} + \frac{1}{r} + \cdots + \frac{1}{r} = \frac{n}{r}\)
Therefore, the total internal resistance is:
\(\displaystyle R_{\text{internal}} = \frac{r}{n}\)
When a load with resistance (R) is connected across the parallel combination of cells, the total current (I) supplied by the combination is the sum of the currents from each cell. Ohm’s law gives us:
\(\displaystyle I = \frac{E_{\text{total}}}{R + R_{\text{internal}}}\)
Substituting (\(\displaystyle E_{\text{total}} = E\)) and (\(\displaystyle R_{\text{internal}} = \frac{r}{n})\):
\(\displaystyle I = \frac{E}{R + \frac{r}{n}}\)
The current from each cell (Icell) is given by:
\(\displaystyle I_{\text{cell}} = \frac{E}{R + r}\)
The total current (I) is then:
\(\displaystyle I = n \times I_{\text{cell}} = n \times \frac{E}{R + r}\)
Also Read: Electrical Cell
Solved Examples
Problem 1: Three cells of EMF 1.5V, 2.0V, and 2.5V are connected in series. Calculate the equivalent EMF of the combination.
Solution: When cells are connected in series, their EMFs add up directly.
\(\displaystyle \text{Equivalent EMF} (E_{\text{eq}}) = E_1 + E_2 + E_3 \)
Given: \(\displaystyle E_1 = 1.5 \, \text{V} \); \(\displaystyle E_2 = 2.0 \, \text{V} \); \(\displaystyle E_3 = 2.5 \, \text{V} \)
\(\displaystyle E_{\text{eq}} = 1.5 \, \text{V} + 2.0 \, \text{V} + 2.5 \, \text{V} = 6.0 \, \text{V} \)
The equivalent EMF of the combination of cells in series is 6.0V.
Problem 2: Three cells with internal resistances 0.5Ω, 1.0Ω, and 1.5Ω are connected in series. Calculate the equivalent internal resistance of the combination.
Solution: When cells are connected in series, their internal resistances add up directly.
\(\displaystyle \text{Equivalent Internal Resistance} (r_{\text{eq}}) = r_1 + r_2 + r_3 \)
Given: \(\displaystyle r_1 = 0.5 \, \Omega \); \(\displaystyle r_2 = 1.0 \, \Omega \); \(\displaystyle r_3 = 1.5 \, \Omega \)
\(\displaystyle r_{\text{eq}} = 0.5 \, \Omega + 1.0 \, \Omega + 1.5 \, \Omega = 3.0 \, \Omega \)
The equivalent internal resistance of the combination of cells in series is 3.0Ω.
Problem 3: Two cells of EMF 1.5V and 2.0V with internal resistances 0.5Ω and 1.0Ω, respectively, are connected in parallel. Calculate the equivalent EMF and internal resistance of the combination.
Solution: For cells in parallel, the equivalent EMF (Eeq) is given by:
\(\displaystyle E_{\text{eq}} = \frac{E_1 r_2 + E_2 r_1}{r_1 + r_2} \)
And the equivalent internal resistance (\(\displaystyle r_{\text{eq}}\)) is given by:
\(\displaystyle \frac{1}{r_{\text{eq}}} = \frac{1}{r_1} + \frac{1}{r_2} \)
Given:
\(\displaystyle E_1 = 1.5 \), \text{V}, \(\displaystyle\quad r_1 = 0.5 \), \(\displaystyle\Omega \)
\(\displaystyle E_2 = 2.0 \), \text{V}, \(\displaystyle\quad r_2 = 1.0 \), \(\displaystyle\Omega \)
Calculate (\(\displaystyle E_{\text{eq}}\)):
\(\displaystyle E_{\text{eq}} = \frac{(1.5 \times 1.0) + (2.0 \times 0.5)}{0.5 + 1.0} = \frac{1.5 + 1.0}{1.5} = \frac{2.5}{1.5} = 1.67 \, \text{V} \)
Calculate (\(\displaystyle r_{\text{eq}}\)):
\(\displaystyle \frac{1}{r_{\text{eq}}} = \frac{1}{0.5} + \frac{1}{1.0} = 2 + 1 = 3 \)
\(\displaystyle r_{\text{eq}} = \frac{1}{3} = 0.33 \, \Omega \)
The equivalent EMF of the combination of cells in parallel is 1.67V and the equivalent internal resistance is 0.33Ω.
Problem 4: Two cells of EMF 1.5V and 2.5V with internal resistances 0.4Ω and 0.6Ω, respectively, are connected in parallel. Determine the maximum current that can be drawn from the combination.
Solution: The maximum current that can be drawn from the combination is determined by the equivalent EMF and internal resistance, using Ohm’s Law:
\(\displaystyle I_{\text{max}} = \frac{E_{\text{eq}}}{r_{\text{eq}}} \)
Using the formulas from Problem 3:
\(\displaystyle E_{\text{eq}} = \frac{(1.5 \times 0.6) + (2.5 \times 0.4)}{0.4 + 0.6} = \frac{0.9 + 1.0}{1.0} = 1.9 \, \text{V} \)
\(\displaystyle \frac{1}{r_{\text{eq}}} = \frac{1}{0.4} + \frac{1}{0.6} = 2.5 + 1.67 = 4.17 \)
\(\displaystyle r_{\text{eq}} = \frac{1}{4.17} \approx 0.24 \, \Omega \)
\(\displaystyle I_{\text{max}} = \frac{1.9}{0.24} \approx 7.92 \, \text{A} \)
The maximum current that can be drawn from the combination of cells in parallel is approximately 7.92A.
Problem 5: Four cells each with EMF 1.5V and internal resistance 0.5Ω are connected in two parallel pairs, and these pairs are connected in series. Calculate the equivalent EMF and internal resistance of the combination.
Solution: For two cells in parallel:
\(\displaystyle E_{\text{parallel}} = \frac{E_1 r_2 + E_2 r_1}{r_1 + r_2} = \frac{(1.5 \times 0.5) + (1.5 \times 0.5)}{0.5 + 0.5} = 1.5 \, \text{V} \)
\(\displaystyle r_{\text{parallel}} = \frac{r_1 r_2}{r_1 + r_2} = \frac{0.5 \times 0.5}{0.5 + 0.5} = 0.25 \, \Omega \)
Each parallel combination has:
\(\displaystyle E_{\text{parallel}} = 1.5 \, \text{V} \)
\(\displaystyle r_{\text{parallel}} = 0.25 \, \Omega \)
These two parallel combinations are then connected in series:
\(\displaystyle E_{\text{series}} = E_{\text{parallel}} + E_{\text{parallel}} = 1.5 \, \text{V} + 1.5 \, \text{V} = 3.0 \, \text{V} \)
\(\displaystyle r_{\text{series}} = r_{\text{parallel}} + r_{\text{parallel}} = 0.25 \, \Omega + 0.25 \, \Omega = 0.5 \, \Omega \)
The equivalent EMF of the mixed series-parallel combination is 3.0V and the equivalent internal resistance is 0.5Ω.
Problem 6: A load of 5Ω is connected across two cells in series, each with an EMF of 1.5V and internal resistance of 0.2Ω. Calculate the power delivered to the load.
Solution: Equivalent EMF and internal resistance for cells in series:
\(\displaystyle E_{\text{eq}} = 1.5 \, \text{V} + 1.5 \, \text{V} = 3.0 \, \text{V} \)
\(\displaystyle r_{\text{eq}} = 0.2 \, \Omega + 0.2 \, \Omega = 0.4 \, \Omega \)
Total resistance in the circuit:
\(\displaystyle R_{\text{total}} = R_{\text{load}} + r_{\text{eq}} = 5 \, \Omega + 0.4 \, \Omega = 5.4 \, \Omega \)
Current through the circuit:
\(\displaystyle I = \frac{E_{\text{eq}}}{R_{\text{total}}} = \frac{3.0 \, \text{V}}{5.4 \, \Omega} = 0.556 \, \text{A} \)
Power delivered to the load:
\(\displaystyle P = I^2 \times R_{\text{load}} = (0.556 \, \text{A})^2 \times 5 \, \Omega \)
\(\displaystyle P = 0.309 \, \text{A}^2 \times 5 \, \Omega = 1.545 \, \text
{W} \)
The power delivered to the load is 1.545W.
FAQs
What is the difference between cells connected in series and cells connected in parallel?
In a series connection, the cells are connected end-to-end, so the total voltage is the sum of the individual voltages, but the current remains the same through all cells. In a parallel connection, the cells are connected with all positive terminals together and all negative terminals together, resulting in the total current being the sum of the individual currents, but the voltage remains the same across all cells.
What are the advantages of connecting cells in series?
Connecting cells in series increases the total voltage output, which is useful when a higher voltage is needed to power a device. This setup is often used in applications where the voltage requirement exceeds what a single cell can provide, such as in flashlights or certain electronic devices.
What are the benefits of connecting cells in parallel?
Connecting cells in parallel increases the total current capacity and extends the overall battery life. This configuration is beneficial when a device requires more current than a single cell can provide or when longer operating time is needed, such as in large-capacity battery packs for electric vehicles or backup power supplies.
How does the internal resistance of cells affect their performance in series and parallel combinations?
In a series combination, the total internal resistance is the sum of the internal resistances of all cells, which can lead to a significant voltage drop under load and reduce efficiency. In a parallel combination, the effective internal resistance decreases, improving current delivery and reducing the voltage drop, which makes the parallel combination more efficient for high-current applications.
Can you combine cells in both series and parallel configurations?
Yes, cells can be combined in both series and parallel configurations to achieve a desired voltage and current output. This is often done in battery packs for applications requiring both high voltage and high current, such as in electric vehicles or large-scale energy storage systems.
What are some common applications of series and parallel cell combinations?
Series combinations are commonly used in applications like flashlights, remote controls, and certain electronic devices where higher voltage is needed. Parallel combinations are used in applications such as power banks, electric vehicles, and backup power supplies where higher current capacity and longer battery life are essential.
How can you determine the total voltage and current of a combination of cells?
For cells in series, the total voltage is the sum of the individual cell voltages, while the current remains the same as that of a single cell. For cells in parallel, the total current is the sum of the individual cell currents, while the voltage remains the same as that of a single cell. By combining these principles, you can calculate the overall voltage and current for any combination of series and parallel connections.