What is Electrical Resistivity
Electrical resistivity is a concept that tells us how difficult it is for electric current to flow through a material. Electric current is the movement of electric charges, such as electrons, in a circuit. Some materials, like metals, allow electric current to flow easily. These materials are called conductors and they have low resistivity. Other materials, like rubber or glass, do not allow electric current to flow easily. These materials are called insulators and they have high resistivity. There are also some materials, like silicon, that can behave like conductors or insulators depending on certain conditions. These materials are called semiconductors and they have medium resistivity.
The resistivity of a material depends on its structure and composition. For example, metals have many free electrons that can move around and carry electric current. Insulators have very few or no free electrons, so they cannot carry electric current. Semiconductors have some free electrons, but they can also create more by adding impurities or changing the temperature.
The resistivity of a material is measured in ohm meter (Ω⋅m). This unit tells us how much resistance a material has per unit length and cross-sectional area. Resistance is the opposition to the flow of electric current. The higher the resistivity, the higher the resistance. The lower the resistivity, the lower the resistance. We can calculate the resistivity of a material using the formula:
\(\displaystyle\rho = \frac{RA}{l}\)
where \(\displaystyle\rho\) is the resistivity, R is the resistance, A is the cross-sectional area, and l is the length of the material. This formula shows that the resistivity is proportional to the resistance and the cross-sectional area, and inversely proportional to the length. This means that if we increase the resistance or the cross-sectional area, the resistivity will increase. If we increase the length, the resistivity will decrease.
To help you remember the resistivity formula, you can use the mnemonic RAIL. The first letter of each word corresponds to the first letter of each variable in the formula.
Here are some examples of resistivities of different materials at room temperature:
| Material | Resistivity (Ω⋅m) |
| Silver | 1.59 ×10-8 |
| Copper | 1.68 × 10-8 |
| Iron | 9.71 × 10-8 |
| Carbon | 3.5 × 10-5 |
| Silicon | 6.40 × 102 |
| Glass | 1010 to 1014 |
| Rubber | 1013 |
at room temperature.
Resistivity is a measure of how much a material resists the flow of electric current. The lower the resistivity, the easier it is for current to flow. The higher the resistivity, the harder it is for current to flow.
The table is divided into three categories: conductors, semiconductors, and insulators. Conductors are materials that have very low resistivity and can carry electric current well. Examples are silver, copper, iron, etc. Semiconductors are materials that have medium resistivity and can change their conductivity by adding impurities or changing the temperature. Examples are silicon, germanium, etc. Insulators are materials that have very high resistivity and cannot carry electric current well. Examples are glass, rubber, etc.
Resistor color code
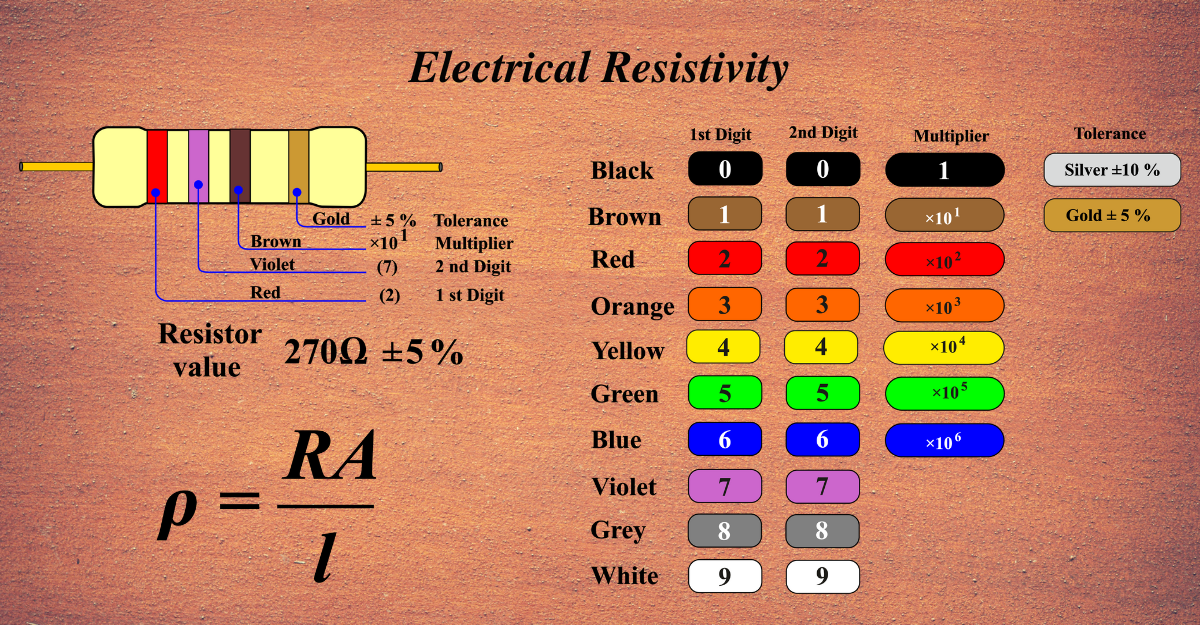
The resistor color code is a system of using colored bands on a resistor to indicate its resistance value and tolerance. The colors represent digits from 0 to 9, and also multipliers and tolerances. The resistor color code usually has four bands, but sometimes five or six bands are used for more precision.
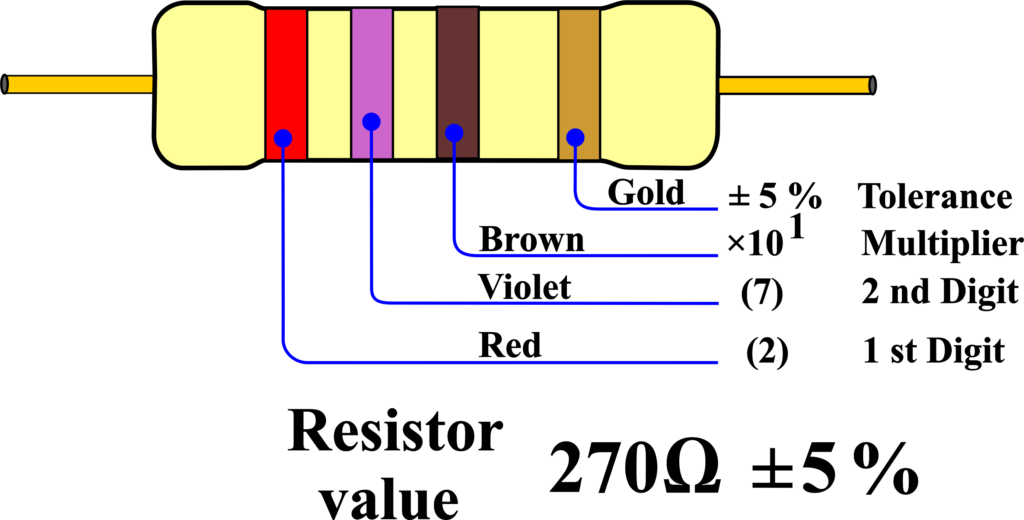
The first two bands indicate the first and second digits of the resistance value. The third band indicates the multiplier, which is the power of ten to multiply the first two digits by. The fourth band indicates the tolerance, which is the percentage of error that the resistor may have from its nominal value. The fifth and sixth bands, if present, indicate the temperature coefficient and the failure rate, respectively.
To read the resistor color code, you need to memorize the color-digit table, or use a mnemonic device to help you remember. For example, you can use the phrase “Big Brown Rabbits Often Yield Great Big Vegetable Gardens” to remember the colors from black to white.
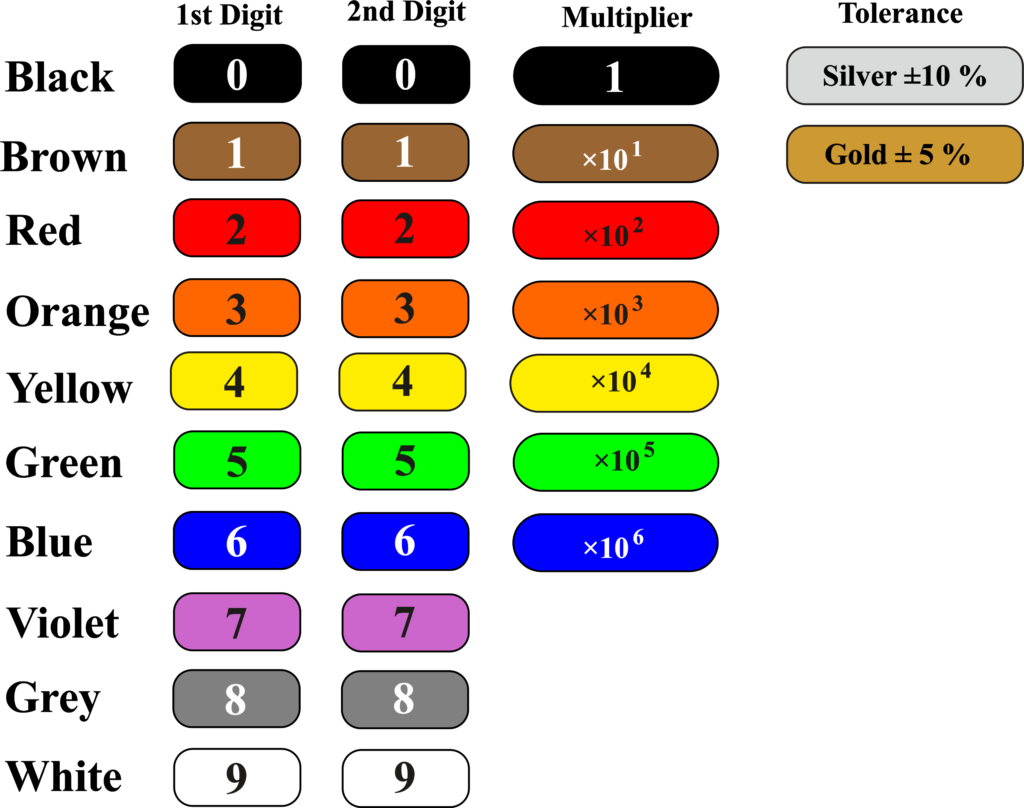
Then, you can use the following steps to find the resistance value and tolerance:
- Hold the resistor with the bands on the left and the tolerance band on the right.
- Identify the colors of the first two bands and write down the corresponding digits.
- Identify the color of the third band and write down the number of zeros to add after the first two digits.
- Identify the color of the fourth band and write down the percentage of tolerance.
For example, if the bands are red, violet, brown, and gold, the resistance value is 27 Ω with a tolerance of ±5%. If the bands are orange, orange, orange, silver, and red, the resistance value is 33 kΩ with a tolerance of ±10% and a temperature coefficient of 50 ppm/°C.
The resistor color code is a simple and convenient way to identify the resistance value and tolerance of a resistor without using a multimeter or a datasheet. However, it can be difficult to read the colors accurately, especially if the resistor is old or damaged. Therefore, it is advisable to always check the resistance value with a multimeter before using a resistor in a circuit.
Temperature dependence of Resistivity
Temperature dependence of resistivity is the phenomenon that describes how the resistivity of a material changes with temperature. Resistivity is a measure of how much a material resists the flow of electric current. The lower the resistivity, the easier it is for current to flow. The higher the resistivity, the harder it is for current to flow.
Different materials have different temperature dependence of resistivity. For most metals, the resistivity increases with temperature. This is because the metal atoms vibrate more when heated, and this creates more obstacles for the free electrons that carry the current. The more collisions the electrons have with the atoms, the more resistance they face, and the higher the resistivity becomes.
For most insulators and semiconductors, the resistivity decreases with temperature. This is because these materials have fewer free electrons at low temperatures, and more free electrons are created when heated. The more free electrons there are, the more current they can carry, and the lower the resistivity becomes.
The temperature dependence of resistivity can be expressed by a formula:
\(\displaystyle \rho_{T} = \rho_{0 }[1 + \alpha (T – T_{0})]\)
where,
- \(\displaystyle\rho_{T}\) is the resistivity at temperature T,
- \(\displaystyle\rho_{0}\) is the resistivity at a reference temperature
- \(\displaystyle T_{0}\), and \(\displaystyle\alpha\) is the temperature coefficient of resistivity, which is a constant that depends on the material.
This formula shows that the resistivity is proportional to the temperature difference \(\displaystyle (T – T_0)\). The sign of \(\displaystyle\alpha\) determines whether the resistivity increases or decreases with temperature. For metals, \(\displaystyle\alpha\) is positive, and for insulators and semiconductors, \(\displaystyle\alpha\) is negative.
The formula shows how the resistivity of a material changes with temperature. For most metals, the resistivity increases with temperature, because the metal atoms vibrate more and create more obstacles for the free electrons that carry the current. For most insulators and semiconductors, the resistivity decreases with temperature, because more free electrons are created by heating the material.
The temperature coefficient of resistivity, \(\displaystyle\alpha\), tells us how much the resistivity changes for a given change in temperature. It is positive for metals and negative for insulators and semiconductors. The higher the absolute value of \(\displaystyle\alpha\), the more sensitive the resistivity is to temperature.
To use the formula, we need to know the resistivity of the material at a reference temperature, usually 20°C, and the temperature coefficient of resistivity for the material. Then, we can plug in the values and calculate the resistivity at any other temperature.
Example: Let’s say we want to find the resistivity of copper at 100°C. We know that the resistivity of copper at 20°C is \(\displaystyle 1.68 \times 10^{-8}\) Ω⋅m$, and the temperature coefficient of resistivity for copper is \(\displaystyle 3.9 \times 10^{-3}\) °C-1. We can use the formula as follows:
\(\displaystyle\rho_{T} = \rho_0 [1 + \alpha (T – T_0)]\)
\(\displaystyle\rho_{100} = (1.68 \times 10^{-8}) [1 + (3.9 \times 10^{-3}) (100 – 20)]\)
\(\displaystyle\rho_{100} = (1.68 \times 10^{-8}) [1.312]\)
\(\displaystyle\rho_{100} = 2.20 \times 10^{-8}\)
So, the resistivity of copper at 100°C is \(\displaystyle 2.20 \times 10^{-8}\) Ω⋅m.
Here are some examples of resistivities and temperature coefficients of different materials at room temperature:
| Material | Resistivity (Ω⋅m) | Temperature Coefficient (°C-1) |
|---|---|---|
| Silver | 1.59 × 10-8 | 3.8 × 10-3 |
| Copper | 1.68 × 10-8 | 3.9 × 10-3 |
| Iron | 9.71 × 10-8 | 5.0 × 10-3 |
| Carbon | 3.5 × 10-5 | -5.0 × 10-4 |
| Silicon | 6.40 × 102 | -7.0 × 10-4 |
| Glass | 1010 to $1014 | -3.0 × 10-5 |
| Rubber | 1013 | -1.0 × 10-5 |
The table shows the resistivity and the temperature coefficient of different materials at room temperature. Resistivity is a measure of how much a material resists the flow of electric current. Temperature coefficient is a measure of how much the resistivity changes for a given change in temperature.
The table is divided into three categories: conductors, semiconductors, and insulators. Conductors are materials that have very low resistivity and can carry electric current well. Examples are silver, copper, iron, etc. Semiconductors are materials that have medium resistivity and can change their conductivity by adding impurities or changing the temperature. Examples are silicon, germanium, etc. Insulators are materials that have very high resistivity and cannot carry electric current well. Examples are glass, rubber, etc.
The resistivity of a material is measured in ohm meter (Ω⋅m). This unit tells us how much resistance a material has per unit length and cross-sectional area. Resistance is the opposition to the flow of electric current. The higher the resistivity, the higher the resistance. The lower the resistivity, the lower the resistance.
Also Read: Electrical Energy and Power
Resistivity In Metals
Metals are like highways for electric current, with electrons zooming through them. But as the metal gets hotter, it’s like the highway gets a bit bumpier. The atoms in the metal start jiggling more vigorously because of the heat, and this makes it harder for the electrons to get through. It’s like trying to drive fast on a road that’s getting increasingly potholed.
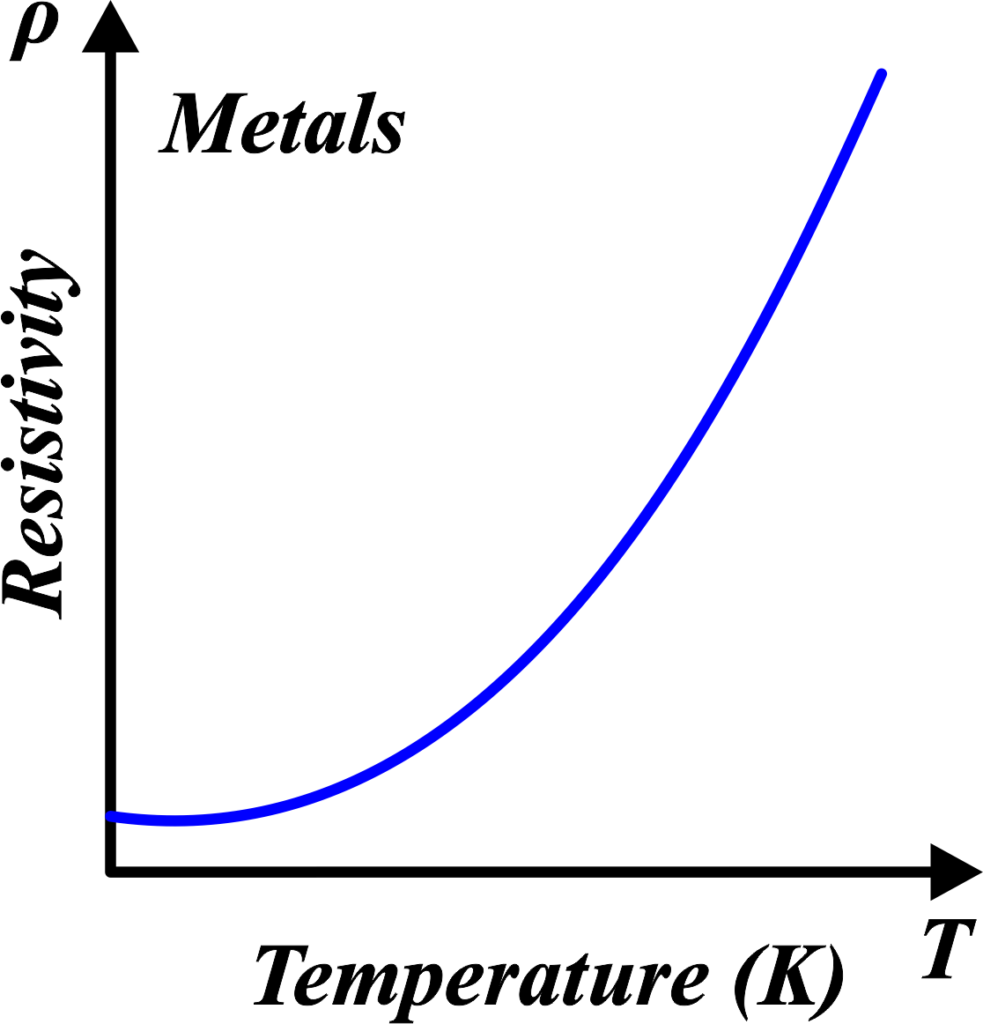
So, as the temperature goes up, the resistivity of the metal also goes up. This is because those jiggling atoms are more likely to get in the way of the electrons, causing more collisions and making it tougher for the current to flow. This relationship is usually linear for metals, meaning if you plotted temperature against resistivity on a graph, you’d get a straight line going up.
Electric current is like a game of tag played by electrons, where they pass on energy from one to another. In materials that conduct electricity, like metals, there’s no “no-go zone” between the energy levels (bands) where electrons can exist. Often, these levels are so close they’re practically high-fiving each other, making it super easy for electrons to jump around and carry current.
Now, metals are pretty chill about letting their electrons go. They have a low “break-up fee” (ionization energy), so electrons can leave the atom without much fuss. When you plug in a metal wire, these free-spirit electrons start moving around, carrying electricity.
But crank up the heat, and the metal atoms start to party harder (vibrate more). This wild dance makes it tough for the electrons to get through the crowd, like trying to walk through a mosh pit. They keep bumping into atoms, losing energy with each bump, which slows down the electric flow. This slow-down is what we call increased resistivity, and it means the metal isn’t as good at conducting electricity anymore.
Metals have this thing called a positive temperature coefficient, which is a fancy way of saying they don’t like heat when it comes to conducting electricity. As the temperature rises, their resistivity goes up predictably, up to a certain point (around 500K). Silver, copper, and gold are classic examples—they’re great conductors, but even they start to struggle when things get too hot.
So, imagine you’re trying to run on a beach as it gets hotter—the sand gets softer, and it’s harder to run. That’s like resistivity increasing with temperature for metals.
Example: Think about the filament in a lightbulb. It’s made of tungsten, a metal with a high melting point. When the filament heats up, its resistivity increases, which is why it glows bright and doesn’t just melt or burn out instantly.
Resistivity In Semiconductors
Imagine a semiconductor as a party venue where electrons are guests. At low temperatures, the venue is pretty chill, and not many guests are dancing (moving around). This is because they’re bound in pairs (covalent bonds), so the resistivity is high, and electricity can’t flow well.

Now, as the temperature rises, the party heats up. The guests (electrons) get more energy to break free from their dance partners (bonds). More and more guests start to move around freely, which means the resistivity drops and the semiconductor becomes a better conductor of electricity. In technical terms, semiconductors like silicon and germanium have fewer charge carriers than metals but more than insulators.
These carriers are generated by the thermal breaking of bonds. So, as the temperature goes up, more covalent bonds break, releasing more electrons, which lowers the resistivity rapidly.
This is the opposite of what happens in metals, where resistivity increases with temperature. In semiconductors, the energy gap between the conduction band (where free electrons can move) and the valence band (where electrons are bound) decreases with an increase in temperature. This allows more electrons to jump up to the conduction band and carry current.
So, in simple words, for semiconductors, a warmer temperature means a livelier party for electrons, leading to better conductivity!
Resistivity In Insulators
In insulators, like plastics, ceramics, or glass, the behavior of resistivity with temperature differs from that of metals. Unlike metals, where resistivity typically increases with temperature, insulators often show the opposite trend: a decrease in resistivity as temperature rises.
This behavior is due to the intrinsic properties of insulating materials. At low temperatures, insulators have very few free electrons available for conduction. As temperature increases, some of the electrons gain enough energy to break free from their bound states and become mobile. This process, known as thermal excitation, increases the number of charge carriers in the material, leading to enhanced conductivity.
Additionally, higher temperatures can also disrupt the ordered arrangement of atoms in the insulator’s lattice structure. This disruption can create more pathways for charge carriers to move through, further reducing resistivity.
Overall, in insulators, the increase in the number of charge carriers and the disruption of the lattice structure due to temperature rise contributes to a decrease in resistivity with increasing temperature. This behavior contrasts with that of metals and is an important concept to understand in the study of electrical conductivity and material properties.
FAQs
How does temperature affect the resistivity of a material?
Generally, in metals, resistivity increases with temperature due to increased atomic vibrations impeding electron flow. However, in certain materials like semiconductors and insulators, resistivity can decrease as temperature rises due to increased carrier mobility.
Can resistivity vary with the shape of the material?
Yes, resistivity can be influenced by the shape of the material. For example, a longer and thinner wire will have higher resistance compared to a shorter and thicker wire, as it offers more resistance to the flow of current.
Is resistivity the same as resistance?
No, resistivity (measured in ohm-meters) is a property of the material itself, while resistance (measured in ohms) depends on both resistivity and the geometry of the material.
What factors affect the resistivity of a material?
Several factors influence resistivity, including temperature, impurities, crystal structure, and material composition. For example, adding impurities to a semiconductor can alter its resistivity.
Can resistivity be negative?
No, resistivity is a positive scalar quantity. However, the concept of negative resistivity can be observed in certain non-linear materials under specific conditions, such as in plasmas or in the presence of magnetic fields.
Why are some materials better conductors than others?
Materials with lower resistivity values are better conductors because they offer less resistance to the flow of electric current. Metals like copper and silver are excellent conductors due to their low resistivity values.
How does resistivity relate to electrical conductivity?
Resistivity and electrical conductivity are inversely related. Materials with low resistivity have high conductivity, meaning they allow electric current to flow easily, while materials with high resistivity have low conductivity.