The concept of heat—how hot or cold something is—has intrigued humans for centuries. Even ancient civilizations noticed the effects of temperature on their surroundings. However, finding precise ways to measure heat posed a challenge.
Ancient Greeks and Chinese likely noticed temperature changes, but they didn’t have specific methods for measuring heat. Their observations laid the groundwork for later developments.
During the Renaissance, scientists and inventors began developing methods to measure temperature more accurately. Proposed using the freezing point of water as a reference for temperature measurement. He suggested measuring temperatures relative to this freezing point.
Recognized the need for two fixed points to create a temperature scale. He chose both Hooke’s freezing point and the boiling point of water as reference points. This allowed interpolation between them.
In the 19th century, scientists studying gas behavior observed that volume changes with temperature. They discovered that gases would theoretically contract to zero volume at a specific temperature—absolute zero. This led to the concept of the Kelvin scale, where absolute zero is the lowest possible temperature.
Santorio Santorio, an Italian physician, invented the sealed liquid thermometer. He observed how liquid moved up a glass tube as it expanded or contracted due to temperature changes. However, this early thermometer lacked precise units.
Fahrenheit began manufacturing thermometers using alcohol and later mercury. Mercury was ideal due to its linear response to temperature changes over a wide range. Fahrenheit’s scale used fixed points—the freezing and boiling points of water—to create a practical temperature scale.
These sensors take advantage of the differential expansion of two bonded metal strips. They are used in thermostats and gas grills, although their accuracy is relatively low.
What is Heat?
Heat is a form of energy that is associated with the movement of atoms and molecules in a substance. It is transferred between bodies or systems due to a temperature difference. Heat flows naturally from a hotter object to a cooler one until thermal equilibrium is reached.
Suppose ice melts, it absorbs heat from its surroundings. This process is an example of heat transfer leading to a phase change. The heat energy causes the molecules in the ice to vibrate more vigorously, breaking the hydrogen bonds and holding them in a solid structure. As the ice absorbs more heat, it eventually gains enough energy to transition into a liquid state, becoming water. Similarly, a bonfire releases heat through combustion, which is a chemical reaction between the fuel (wood) and oxygen from the air. The reaction releases energy in the form of heat and light.


The heat from the bonfire can be felt even from a distance because it is transferred through radiation, which does not require a medium to travel. The heat radiated by the bonfire can be felt by the skin and causes the surrounding air to become warmer, which can then transfer heat to nearby objects through convection and conduction.
The SI unit of heat is the joule (J). However, heat is also commonly measured in calories (cal), where 1 calorie is the amount of heat required to raise the temperature of 1 gram of water by 1°C.
Heat Transfer
Heat transfer is the process by which thermal energy moves from a hotter object to a cooler one until both reach thermal equilibrium. There are three primary modes of heat transfer: conduction, convection, and radiation.
- Convection is the transfer of heat by the physical movement of a fluid (liquid or gas). This can be natural convection, driven by buoyancy forces, or forced convection, driven by external means like a pump or fan.
- Conduction is the transfer of heat through a material without any movement of the material itself. It occurs due to the collision and diffusion of molecules within a solid, liquid, or gas.
- Radiation is the transfer of heat through electromagnetic waves. It does not require a medium and can occur in a vacuum.
Convection
Convection is the process of heat transfer that occurs in fluids (liquids and gases) due to the movement of the fluid itself. This movement is caused by differences in temperature and density within the fluid, leading to the transfer of heat from one part to another.
When a fluid is heated, the particles gain energy and move faster. This causes the heated portion of the fluid to expand, making it less dense than the surrounding cooler fluid. Due to gravity, the denser, cooler fluid sinks, and the less dense, warmer fluid rises. This movement creates a cycle known as a convection current.
There are two main types of convection:
- Natural Convection
- Forced Convection
Natural Convection
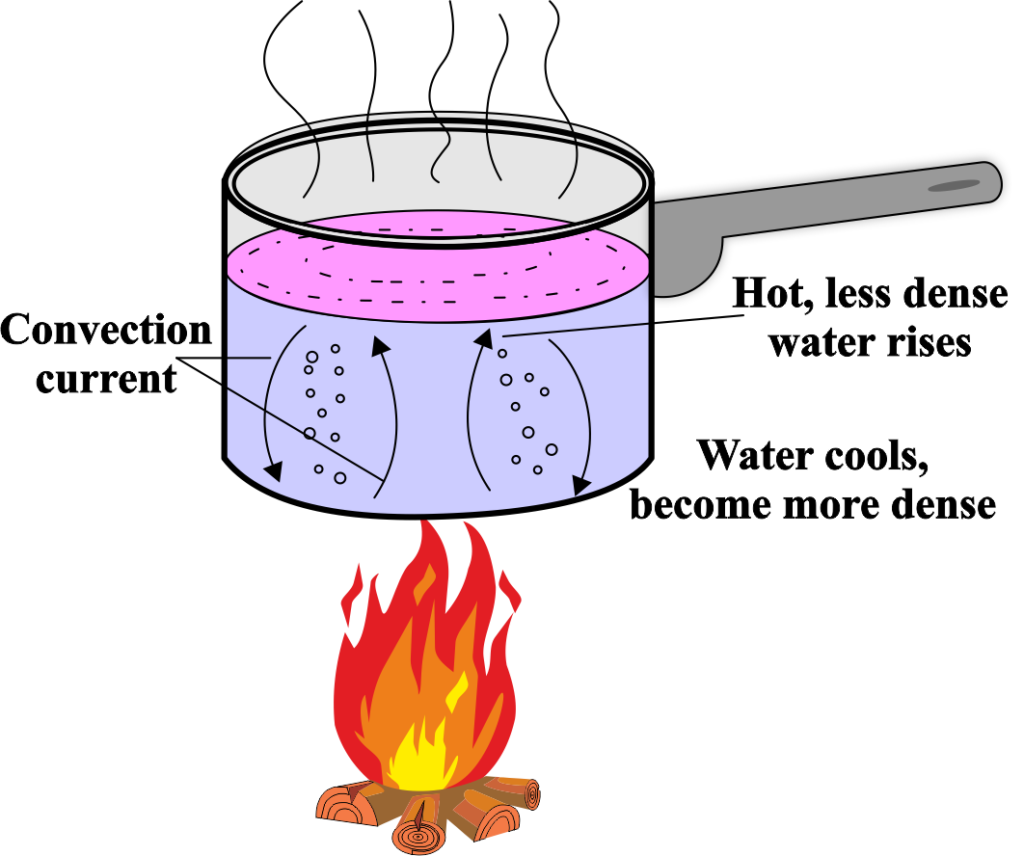
Natural convection, also known as free convection, occurs when the movement of the fluid is caused by natural means, such as the buoyant force created by temperature differences within the fluid.
For example, the warm air rising above a radiator and the circulation of water in a pot being heated on a stove are instances of natural convection.
Factors Affecting Natural Convection: Several factors influence the rate and efficiency of natural convection:
- Temperature Gradient: The greater the difference in temperature between the warm and cool areas of the fluid, the stronger the convection currents.
- Fluid Properties: The density, viscosity, and specific heat capacity of the fluid affect how easily it can flow and transfer heat.
- Gravitational Field: Natural convection relies on gravity to create the buoyant forces necessary for fluid movement.
The rate of heat transfer in natural convection can be described using a similar formula to forced convection, but the heat-transfer coefficient will be different due to the absence of external forces.
Examples
- The movement of air in the atmosphere creates winds and weather patterns.
- The vast movements of water in the oceans (Ocean Currents) regulate climate by transferring heat around the globe.
- Radiators in a room heat the air, which rises and circulates to warm the entire space.
Understanding natural convection is crucial for many applications, including environmental science, engineering, and technology design. It helps us predict weather patterns, design efficient cooling systems, and understand natural phenomena like the formation of sea breezes. Natural convection is a fascinating topic because it combines principles of thermodynamics, fluid dynamics, and heat transfer in a way that we can observe in many everyday situations.
Forced Convection
Forced convection is a type of heat transfer that occurs in fluids (liquids and gases) when the fluid is forced to move over a surface or through a fluid medium by an external source such as fans, pumps, or blowers. Unlike natural convection, where the fluid motion is due to buoyancy forces, forced convection requires an external mechanism to move the fluid.
In forced convection, the external force overcomes the natural viscosity of the fluid, allowing for more efficient mixing and heat transfer. The fluid absorbs heat from a hot surface or dissipates heat to a cooler surface, depending on the application.
Factors Affecting Forced Convection The efficiency of forced convection is influenced by several factors:
- Velocity of the Fluid: Higher velocities increase the rate of heat transfer.
- Properties of the Fluid: Density, viscosity, specific heat, and thermal conductivity play significant roles.
- Shape and Roughness of the Surface: These affect the flow patterns and heat transfer rate.
- Temperature Difference: The greater the temperature difference between the fluid and the surface, the higher the rate of heat transfer.
The rate of heat transfer in forced convection can be described by Newton’s law of cooling:
\(\displaystyle\begin{equation}\label{eqn:1}\boxed{\boldsymbol{ P = \frac{dQ}{dt} = hA(T_s – T_f) }} \end{equation}\)
- P is the rate of heat transfer,
- \(\displaystyle\frac{dQ}{dt} \) is the heat transfer per unit time,
- h is the convection heat transfer coefficient,
- A is the surface area,
- Ts is the temperature of the surface,
- Tf is the temperature of the fluid.
The heat-transfer coefficient (h) is a critical factor and depends on the fluid properties and flow conditions.
Applications
- Forced convection is used in radiators, air conditioners, and refrigerators.
- It is applied in cooling electronic devices, chemical reactors, and heat exchangers.
- Ovens and stoves often use fans to distribute heat evenly.
Forced convection is a vital concept in engineering and physics because it allows for controlled and efficient heat transfer in various applications. Understanding the principles behind it is essential for designing systems that rely on maintaining specific temperatures or for processes that require heating or cooling.
Also Read: Temperature
Conduction
Conduction is the process of heat transfer through direct contact between atoms and molecules within a material or between different materials. Conduction occurs when there is a temperature difference within a material or between materials in contact. Heat flows from the higher temperature region to the lower temperature region. This flow continues until thermal equilibrium is reached, meaning that the temperature becomes uniform throughout the material.
At the microscopic level, conduction results from the kinetic energy transfer between adjacent atoms and molecules. When one molecule vibrates due to thermal energy, it collides with its neighbor, transferring some of its energy. This chain of energy transfer results in the flow of heat.
Factors Affecting Conduction
- Material: Different materials have different abilities to conduct heat, known as thermal conductivity.
- Temperature Gradient: The heat transfer rate is proportional to the temperature difference across the material.
- Cross-sectional Area: The larger the area through which heat is being transferred, the greater the rate of conduction.
- Thickness: The thinner the material, the faster the rate of heat transfer.
The rate of heat transfer (Q) through conduction can be calculated using Fourier’s law:
\(\displaystyle\begin{equation}\label{eqn:2}\boxed{\boldsymbol{ Q = -kA\frac{dT}{dx} }} \end{equation}\)
Or
\(\displaystyle\begin{equation}\label{eqn:6}\boxed{\boldsymbol{\frac{Q}{t}=\frac{{kA\left( {{{T}_{2}}-{{T}_{1}}} \right)}}{d} }} \end{equation}\)
- Q is the heat transfer per unit time,
- k is the thermal conductivity of the material,
- A is the cross-sectional area,
- \(\displaystyle\frac{dT}{dx} \) is the temperature gradient along the direction of heat transfer.
Examples
- When a metal pan is heated on a stove, the heat is conducted from the bottom of the pan to its contents.
- Materials with low thermal conductivity are used to prevent heat from escaping or entering buildings.
- Conductive materials are used to dissipate heat from electronic components to prevent overheating.
Steady-State Conduction
Steady-state conduction refers to a condition in which the temperature distribution (and thus the heat transfer) within a solid is constant over time. This means that although heat is being transferred through the material, the amount of heat entering any section of the material is equal to the amount of heat leaving it, resulting in no net change in temperature at any point within the material over time.
Characteristics of Steady-State Conduction
- Constant Temperature Gradient: In steady-state conduction, the temperature gradient within the material does not change with time.
- Unchanging Rate of Heat Transfer: The rate at which heat is conducted through the material remains constant.
- Thermal Equilibrium: Each section of the material has reached a thermal equilibrium where the heat flow is balanced.
Understanding steady-state conduction is crucial for solving practical problems involving heat transfer. For example, it helps in designing insulation for buildings, creating thermal management systems for electronic devices, and engineering various heat-exchanging devices.
Examples
- In an insulated pipe carrying hot fluid, the steady state is achieved when the heat conducted through the pipe wall is constant, ensuring minimal energy loss.
- The walls of a house during a cold day reach a steady state when the heat conducted from the inside to the outside is constant, which is essential for maintaining a comfortable indoor temperature.
Transient conduction, also known as unsteady-state conduction, occurs when the temperature within a material changes with time. Unlike steady-state conduction, where temperatures at any point in the material do not change over time, transient conduction involves time-dependent temperature variations.
Transient Conduction
Transient conduction happens when a material initially at a uniform temperature is suddenly exposed to a different temperature at its boundary. This could be due to a sudden change in the surroundings or because of an applied heat source or sink. The heat will begin to diffuse into or out of the material, causing the temperatures within the material to change until a new steady state is reached.
Factors Affecting Transient Conduction
- Thermal Conductivity: The rate at which heat can be conducted through the material.
- Specific Heat: The amount of heat required to raise the temperature of a unit mass of the material by one degree.
- Density: The mass per unit volume of the material.
- Initial and Boundary Conditions: The initial temperature distribution and the temperatures imposed at the boundaries of the material.
The transient conduction can be described by the heat equation, which is a partial differential equation:
\(\displaystyle\begin{equation}\label{eqn:4}\boxed{\boldsymbol{ \frac{\partial T}{\partial t} = \alpha \nabla^2 T }} \end{equation}\)
- α is the thermal diffusivity of the material \(\displaystyle\alpha = \frac{k}{\rho c_p} \),
- ρ is the density,
- cp is the specific heat at constant pressure,
- (∇2) is the Laplacian operator representing the spatial second derivatives.
Examples
- When a hot metal object is quenched in water, the surface temperature drops rapidly, and heat conducts away from the surface over time.
- When food is placed in an oven, the outer layers heat up first, and the heat gradually moves inward.
Transient conduction is a complex phenomenon because it requires an understanding of how heat moves through materials over time. It’s essential for predicting how temperatures will change in a system, which is crucial for many engineering applications, such as thermal management in electronics, material processing, and even the culinary arts.
Radiation
Radiation is a method of heat transfer that does not require any medium. It can occur in a vacuum and is the primary way that energy from the sun reaches the Earth. This mode of heat transfer is based on electromagnetic waves or photons. Radiation occurs when a body emits energy in the form of electromagnetic waves or particles. These waves carry energy away from the emitting body and can transfer that energy when they interact with another body.
Characteristics of Radiation
- Radiation includes a wide range of wavelengths, from long-wave infrared to short-wave gamma rays. Visible light is also a form of radiation.
- All electromagnetic waves travel at the speed of light in a vacuum.
- Radiation can transfer energy through space, unlike conduction and convection which require a medium.
The rate at which a body emits or absorbs radiation can be described by the Stefan-Boltzmann law:
\(\displaystyle\begin{equation}\label{eqn:5}\boxed{\boldsymbol{ P = \sigma e A T^4 }} \end{equation}\)
Where:
- P is the power radiated,
- σ is the Stefan-Boltzmann constant \(\displaystyle 5.67 \times 10^{-8} \text{W/m}^2\text{K}^4\),
Examples
- The Earth receives heat and light from the sun through radiation.
- Uses microwaves, a form of radiation, to heat food.
- Used in medical imaging, is a higher-energy form of radiation.
Solve Examples
Example 1: A metal rod of length 2 meters and cross-sectional area 0.02 m² has a temperature difference of 100°C between its ends. If the thermal conductivity of the metal is (200 W/mK), calculate the rate of heat transfer through the rod.
Solution: Using the formula for heat conduction:
\(\displaystyle Q = \frac{k \cdot A \cdot \Delta T}{L} \)
Plugging in the values, we get:
\(\displaystyle Q = \frac{200 \, \text{W/mK} \times 0.02 \, \text{m²} \times 100°C}{2 \, \text{m}} \)
\(\displaystyle Q = 2000 \, \text{W} \)
So, the rate of heat transfer through the rod is (2000 W).
Example 2: A glass of hot water at (80°C) is left to cool in a room at (20°C). If the convection coefficient is (15 W/m²K), calculate the rate of heat loss from the water to the surroundings.
Solution: Using Newton’s law of cooling:
\(\displaystyle Q = h \cdot A \cdot \Delta T \)
Given (∆T = 80°C – 20°C = 60°C ) let’s assume the surface area of the glass is (0.1 m²).
\(\displaystyle Q = 15 \, \text{W/m²K} \times 0.1 \, \text{m²} \times 60°C \)
\(\displaystyle Q = 90 \, \text{W} \)
So, the rate of heat loss from the water to the surroundings is (90 W).
Example 3: A black body radiator has a surface area of (0.5 m²) and emits radiation at a rate of (500W/m²). Calculate the total power emitted by the radiator.
Solution: The total power emitted by the radiator is given by:
\(\displaystyle P = \sigma \cdot A \cdot T^4 \)
where (P) is the power emitted, (σ) is the Stefan-Boltzmann constant (5.67 × 10-8, W/m²K⁴), (A) is the surface area, and (T) is the temperature of the radiator in Kelvin.
Let’s assume the temperature of the radiator is (400 K).
\(\displaystyle P = (5.67 \times 10^{-8} \, \text{W/m²K⁴}) \times 0.5 \, \text{m²} \times (400 \, \text{K})^4 \)
\(\displaystyle P \approx 1440 \, \text{W} \)
So, the total power emitted by the radiator is approximately (1440 W).
Example 4: A metal plate of thickness (0.1 m) and thermal conductivity (150 W/mK) is exposed to a fluid flow on one side and a temperature of (100°C) on the other side. If the convection coefficient is (25 W/m²K), calculate the rate of heat transfer through the plate.
Solution: The rate of heat transfer through the plate involves both conduction and convection. We can calculate the rate of heat transfer by conduction through the plate and then add the rate of heat transfer by convection on the other side.
\(\displaystyle Q_{\text{cond}} = \frac{k \cdot A \cdot \Delta T}{L} \)
\(\displaystyle Q_{\text{cond}} = \frac{150 \, \text{W/mK} \times A \times 100°C}{0.1 \, \text{m}} \)
For simplicity, let’s assume (A = 1 m²).
\(\displaystyle Q_{\text{cond}} = 150000 \, \text{W} \)
The rate of heat transfer by convection is given by
\(\displaystyle Q_{\text{conv}} = h \cdot A \cdot \Delta T \).
\(\displaystyle Q_{\text{conv}} = 25 \, \text{W/m²K} \times 1 \, \text{m²} \times 100°C \)
\(\displaystyle Q_{\text{conv}} = 2500 \, \text{W} \)
Therefore, the total rate of heat transfer through the plate is
\(\displaystyle Q_{\text{total}} = Q_{\text{cond}} + Q_{\text{conv}} = 150000 \, \text{W} + 2500 \, \text{W} = 152500 \, \text{W} \)
Example 5: A steel plate with emissivity (0.8) and surface area (0.5 m²) is at (600°C). Calculate the rate at which it emits radiation.
Solution: The rate at which the steel plate emits radiation can be calculated using the Stefan-Boltzmann law:
\(\displaystyle P = \varepsilon \cdot \sigma \cdot A \cdot T^4 \)
Given (\(\displaystyle\varepsilon = 0.8 \)), (A = 0.5 m²), and (T = 600°C = 873 K):
\(\displaystyle P = (0.8) \cdot (5.67 \times 10^{-8} \, \text{W/m²K⁴}) \cdot (0.5 \, \text{m²}) \cdot (873 \, \text{K})^4 \)
\(\displaystyle P \approx 1159.76 \, \text{W} \)
So, the rate at which the steel plate emits radiation is approximately (1159.76 W).
FAQs
Why does metal feel colder to the touch compared to wood at the same temperature?
Metals are better conductors of heat than wood. When you touch metal, it conducts heat away from your hand faster, making it feel colder. Wood, being a poorer conductor, doesn’t transfer heat away from your hand as quickly, so it feels warmer.
Can heat transfer occur in a vacuum?
Yes, heat transfer can occur in a vacuum through radiation. Unlike conduction and convection, which require a medium like solid, liquid, or gas, radiation can transfer heat energy through space. This is how the Sun’s energy reaches the Earth.
Why does the handle of a spoon left in a hot cup of tea become hot, while the end of the tea remains cool?
This phenomenon is due to conduction. The heat from the tea is transferred to the spoon’s handle through conduction. As the handle conducts heat, it becomes hot. However, since the tea at the end of the spoon cools down the spoon by absorbing its heat, that part remains relatively cool.
How does a thermos flask keep hot liquids hot and cold liquids cold?
A thermos flask minimizes heat transfer through conduction, convection, and radiation. It achieves this through a vacuum-sealed double-wall construction. The vacuum between the walls prevents heat transfer via conduction and convection, while the reflective surface on the inner wall reduces radiation heat transfer. This effectively maintains the temperature of the contents for an extended period.
Why does blowing on hot soup cool it down?
Blowing on hot soup increases the rate of evaporation, which is a cooling process. When you blow on the soup, you increase the flow of air over its surface, facilitating the evaporation of hot liquid molecules. As these molecules evaporate, they take away heat energy from the soup, effectively cooling it down.

Very good one
Keep up the good works