Heat Capacity

The story of the transformer begins in the 19th century. The principle of electromagnetic induction, which is the science behind transformers, was discovered by Michael Faraday in 1831. However, it wasn’t until the 1880s that the first practical transformers were developed.

In the early 1880s, inventors in Hungary created what we might consider the first versions of the modern transformer. These were used in experimental and commercial systems to prove that it was possible to transfer electrical energy efficiently over long distances.

In 1885, an American inventor named William Stanley built a transformer that could be used reliably in commercial applications. His design was based on earlier work but made the transformer easier to produce and adjust for practical use.

The first time a transformer was used in a modern AC power system was in Great Barrington, Massachusetts, in 1886. This event marked the beginning of the widespread use of alternating current for electrical power distribution.

From those early days, the transformer has become an essential part of our electrical systems. It’s used everywhere, from the power lines that bring electricity to our homes to the chargers that power our phones.

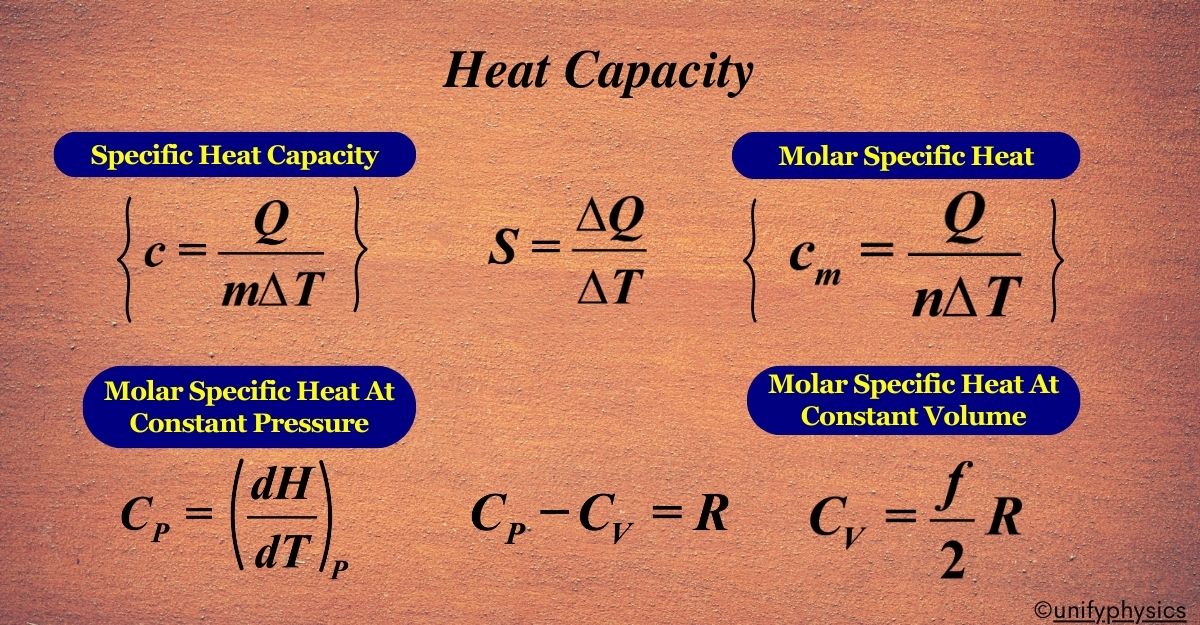
What is Heat Capacity

Imagine you have a big container of water and a small cup of water, and you want to make both equally warm. You’ll notice that you need to heat the big container for a longer time compared to the small cup to achieve the same temperature increase. This is because the big container has a higher heat capacity.

Heat capacity is the amount of heat energy required to raise the temperature of a substance by one degree Celsius. It’s an extensive property, meaning it depends on the amount of substance present.

The heat capacity (S) of an object is the ratio of the heat (ΔQ) added to or removed from the object to the resulting temperature change (ΔT):

\(\displaystyle S = \frac{\Delta Q}{\Delta T} \)

In physics terms, heat capacity is the amount of heat energy required to raise the temperature of an object by one degree Celsius (°C). It’s like the “thermal stamina” of an object—the higher the heat capacity, the more heat energy it can absorb without getting significantly hotter.

Examples: 1) The ocean takes longer to heat up and cool down compared to land, which is why coastal areas have milder climates. 2) A thick cast-iron skillet takes longer to heat up than a thin aluminum pan because it has a higher heat capacity.

Understanding heat capacity helps us in many ways, from designing better cookware to predicting climate patterns. So next time you’re at the beach, think about why the sand gets hot so quickly, while the water stays cool—it’s all about heat capacity!

Specific Heat Capacity (s)

Imagine you’re at the beach on a sunny day. You’ll notice that the sand gets hot very quickly, while the water takes much longer to warm up. This difference is due to a property called specific heat capacity.

Specific heat capacity is a measure of how much heat energy a substance can absorb before its temperature rises by one degree Celsius.

It’s like a “thermal buffer” that tells us how resistant a substance is to changing temperature.

Different materials have different specific heat capacities, which is why some things heat up or cool down faster than others. For example, metals usually have a low specific heat capacity, so they get hot quickly. Water, on the other hand, has a high specific heat capacity, so it takes a lot of energy to raise its temperature. The formula for specific heat capacity (c) is:

\(\displaystyle\begin{equation}\label{eqn:1}\boxed{\boldsymbol{ c = \frac{Q}{m \Delta T}}} \end{equation}\)

• (Q) is the heat energy added (in joules),
• (m) is the mass of the substance (in grams),
• (ΔT) is the change in temperature (in degrees Celsius).

Example: Let’s say you’re heating a pot of water on the stove. The specific heat capacity of water tells you how much heat you need to add to the water to get it to the temperature you want for your tea or coffee.

Molar Specific Heat Capacity

”Molar-specific heat capacity is the amount of heat required to raise the temperature of one mole of a substance by one degree Celsius.”

It’s a way to compare how different substances heat up if we have the same number of particles (or moles) of each. A mole is like a fixed number of particles, just like a dozen is 12 of something. By using moles, scientists can compare substances fairly because they’re looking at the same number of molecules or atoms. The formula for molar specific heat capacity (C_m) is:

\(\displaystyle\begin{equation}\label{eqn:2}\boxed{\boldsymbol{C_m = \frac{Q}{n \Delta T} }} \end{equation}\)

• (n) is the number of moles,

When you heat a pot of water, the molar-specific heat capacity tells you how much energy you need for every mole of water molecules to get the water boiling. So, molar-specific heat capacity is all about understanding how much heat energy a “mole” of any substance needs to warm up. It’s a key concept that helps us figure out how to efficiently heat and cool materials, and even how to store energy.

Types of Molar Specific Heat Capacity

There are two types of molar-specific heat capacities:

• C_p: Molar specific heat capacity at constant pressure.
• C_v: Molar specific heat capacity at constant volume.

Molar Specific Heat at Constant Pressure(C_p)

Imagine you’re blowing up a balloon on a cool morning. As you blow air into the balloon, it expands. If you were to heat the balloon, it would expand even more, but the pressure inside would stay the same because the balloon’s skin stretches. This is similar to what happens to gases at constant pressure.

C_p stands for the molar-specific heat capacity of a substance when the pressure doesn’t change. It tells us how much heat energy is needed to raise the temperature of one mole of a substance by one degree Celsius, without changing the pressure.

In many real-life situations, like the atmosphere or an open container, the pressure stays relatively constant. So, C_p gives us a realistic idea of how substances behave in these conditions. The formula for C_p is:

\(\displaystyle C_p = \left( \frac{dH}{dT} \right)_P \)

• (dH) is the change in enthalpy (heat content),
• (dT) is the change in temperature,
• The subscript (P) indicates that the pressure is constant.

Enthalpy and C_p

Enthalpy (H) is a measure of the total energy of a system, including both internal energy and energy required to displace the surroundings to accommodate changes in the system’s volume, such as in chemical reactions at constant pressure. At constant pressure, any heat change in the system is equal to the change in enthalpy.

When you heat a gas at constant pressure, it expands. This expansion does work on the surroundings because the gas pushes against the atmospheric pressure. Now, the heat you add doesn’t just increase the internal energy of the gas (which would increase its temperature); some of that heat also does the work of expansion.

Here’s where enthalpy comes into play. Enthalpy is a measure that combines the internal energy of the system plus the work done by the system when it expands. So, when we talk about C_p, we’re looking at how much heat is needed to raise the temperature of one mole of a substance by one degree Celsius at constant pressure, taking into account both the increase in internal energy and the work done during expansion.

In essence, C_p gives us a more complete picture of the heat required at constant pressure because it includes the energy needed for both heating the substance and doing the work of expansion. This is why C_p is always greater than C_v (molar-specific heat at constant volume), where no work is done because the volume doesn’t change.

For an ideal gas, the relationship between C_p and the molar-specific heat at constant volume (C_v) is given by Mayer’s relation:

\(\displaystyle\begin{equation}\label{eqn:3}\boxed{\boldsymbol{C_p – C_v = R }} \end{equation}\)

where ( R ) is the universal gas constant. This equation is derived from the first law of thermodynamics and the definition of enthalpy (H), which is a state function that reflects the heat content of a system at constant pressure.

For different types of gases, C_p can be further related to the degrees of freedom of the gas molecules:

• For a monatomic gas (like helium or neon), which has three translational degrees of freedom, C_p is given by:

\(\displaystyle Cp = \frac{5}{2}R \)

• For a diatomic or linear molecule gas (like nitrogen or oxygen), which has five degrees of freedom (three translational and two rotational), C_p is:

\(\displaystyle Cp = \frac{7}{2}R \)

• For a nonlinear molecule gas (like methane), which has six degrees of freedom (three translational and three rotational), C_p is:

\(\displaystyle Cp = 4R \)

These formulas are based on the equipartition theorem, which states that each degree of freedom contributes \(\displaystyle\frac{1}{2}RT \) to the internal energy on a molar basis at thermal equilibrium. When you heat water in an open pot, the pressure above the water stays the same (it’s just atmospheric pressure). The amount of heat you need to add to increase the temperature of the water is related to the water’s C_p.

When you heat water in an open pot, the pressure above the water stays the same (it’s just atmospheric pressure). The amount of heat you need to add to increase the temperature of the water is related to the water’s C_p.

So, C_p is all about understanding how much heat energy a substance can take in before it gets hotter, while the pressure around it stays the same. The values of (C_p) and (C_v) differ because work is done by the system at constant pressure when it expands upon heating, which does not occur at constant volume.

Molar specific heat capacity at constant volume (C_v)

Imagine you have a sealed, rigid container that doesn’t expand or contract. Inside this container is a gas. When you heat the gas, its pressure might increase, but the volume stays the same because the container doesn’t allow it to expand. This is what we mean by “constant volume.”

C_v is the amount of heat energy needed to raise the temperature of one mole of a substance by one degree Celsius, without any volume change. Since the volume doesn’t change, no work is done on or by the gas during heating.

C_v is crucial for understanding how substances behave when they’re heated in a closed system. It’s a key concept in thermodynamics that helps us predict how much energy is required to change the temperature of a substance when it’s not allowed to expand. The formula for C_v is:

\(\displaystyle C_v = \frac{Q}{n \Delta T} \)

If you have a can of soup and you heat it on the stove, the soup’s temperature increases, but the can’s volume doesn’t change. The energy you’re adding goes only into increasing the temperature, not doing any work to expand the volume.

Internal Energy and C_v

At constant volume, the heat added to the system is directly related to the increase in its internal energy because there’s no work done (work is related to volume change). So, C_v gives us a direct measure of how much the internal energy of a substance will change with temperature when the volume is held constant.

At constant volume, when you add heat to a substance, all of that energy goes into increasing the speed of the molecules—none of it is used for expansion. This increase in molecular speed means an increase in the substance’s internal energy.

The formula \(\displaystyle C_{v} = \frac{Q}{n\Delta T} \) shows that C_v is directly proportional to the change in internal energy (ΔU) for an ideal gas, because (ΔU) is equal to (Q) when the volume is constant. In other words, C_v tells us how much the internal energy of a mole of gas will increase when the temperature is raised by one degree Celsius, without allowing the gas to expand.

This relationship is particularly straightforward for ideal gases, where the internal energy is purely kinetic and depends only on temperature, not volume or pressure. C_v helps us understand the energy required to heat a substance in a closed system. It’s like knowing how much gas you need to fill your car when the tank is a fixed size—you’re only filling it up, not expanding it.

The relationship between the change in internal energy (ΔU) and the molar specific heat at constant volume (C_v) for a monatomic ideal gas, which leads to the equation \(\displaystyle C_v = \frac{3}{2}R \).

The first law of thermodynamics states that the change in internal energy (ΔU) of a system is equal to the heat added to the system (Q) minus the work done by the system (W):

\(\displaystyle \Delta U = Q – W \)

At constant volume (ΔV=0), no work is done by the gas because work (W) is defined as:

\(\displaystyle W = P \Delta V \)

Therefore, at constant volume, (W = 0) and the first law simplifies to:

\(\displaystyle \Delta U = Q_v \)

The heat added at constant volume (Q_v) can be expressed in terms of the molar-specific heat at constant volume (C_v) and the temperature change (ΔT):

\(\displaystyle Q_v = nC_v\Delta T \)

Substituting this into the simplified first law gives:

\(\displaystyle \Delta U = nC_v\Delta T \)

For a monatomic ideal gas, the internal energy is purely kinetic and depends on the degrees of freedom (f). Each degree of freedom contributes \(\displaystyle\frac{1}{2}RT \) per mole to the internal energy. A monatomic gas has 3 translational degrees of freedom, so:

\(\displaystyle U = \frac{3}{2}nRT \)

To find (C_v), we differentiate the internal energy with respect to temperature at constant volume:

\(\displaystyle C_v = \left( \frac{\partial U}{\partial T} \right)_V = \frac{3}{2}nR \)

Since (C_v) is the molar-specific heat capacity, we divide by the number of moles (( n )) to get the value per mole:

\(\displaystyle\begin{equation}\label{eqn:5}\boxed{\boldsymbol{ C_v = \frac{3}{2}R}} \end{equation}\)

The equation \(\displaystyle C_v = \frac{3}{2}R \) tells us that for a monatomic ideal gas, the molar specific heat at constant volume is \(\displaystyle\frac{3}{2} \) times the universal gas constant. This is a result of the kinetic theory of gases, which assumes that the particles are point masses with no intermolecular forces and that the energy is purely translational. For polyatomic gases, the relationship would include additional terms to account for rotational and vibrational degrees of freedom.

For an ideal gas, the internal energy is related to temperature and the degrees of freedom (f ) of the gas molecules. The molar-specific heat at constant volume for an ideal gas can be expressed using the degrees of freedom:

\(\displaystyle\begin{equation}\label{eqn:6}\boxed{\boldsymbol{ C_v = \frac{f}{2}R}} \end{equation}\)

where (R) is the universal gas constant, and (f) is the number of degrees of freedom. For a monatomic ideal gas, (f) is 3 (translational degrees of freedom), and for a diatomic gas, (f) is 5 (3 translational and 2 rotational degrees of freedom).

Also Read: Thermodynamic System

Relation between Specific Heat and Molar Specific Heat Capacity

The molar specific heat capacity is related to the specific heat capacity by the molar mass of the substance. Molar mass (M) is the mass of 1 mole of a substance. Think of specific heat capacity as the amount of heat energy needed for a single ‘particle’ of a substance (like a tiny grain of sand) to increase its temperature by one degree. Now, if we want to understand how much energy it takes for a whole ‘beach’ of these particles (which is a mole of them) to increase their temperature, we look at the molar-specific heat capacity.

The connection is like a recipe conversion. If you know how much spice is needed for one serving and you want to cook for a party (a mole of servings), you multiply the spice amount by the number of servings to get the total spice needed. Similarly, to find the molar specific heat capacity, you multiply the specific heat capacity by the molar mass of the substance.

In mathematical terms, the molar specific heat capacity (C_m) is the product of the specific heat capacity (c) and the molar mass (M) of the substance:

\(\displaystyle C_m = c \times M \)

This formula means that if you know how much energy it takes to heat up a tiny amount of a substance (specific heat capacity), you can figure out how much energy it would take to heat up an entire mole of that substance (molar specific heat capacity) by simply multiplying by the molar mass.

If you think of specific heat capacity as the heat needed for a single ‘particle’, and you have a whole bunch of these particles making up a mole, then the total heat for the mole is just the heat for one particle times the number of particles in a mole (which is the molar mass). This derivation shows the direct proportionality between the specific heat capacity and the molar specific heat capacity, scaled by the molar mass of the substance. It’s a simple yet powerful relationship that helps us understand how substances absorb heat on both a small and large scale.

Solved Examples

Problem 1: A copper block of mass 500 g is heated from 20°C to 100°C. The specific heat capacity of copper is 0.385 J/g°C. Calculate the heat required to achieve this temperature change.

Solution:

• Mass (m) = 500 g
• Initial temperature (T_i) = 20°C
• Final temperature (T_f) = 100°C
• Specific heat capacity of copper (c) = 0.385 J/g°C

The heat required (Q) is given by:

\(\displaystyle Q = mc\Delta T \)

where (\(\displaystyle \Delta T = T_f – T_i \)):

\(\displaystyle \Delta T = 100 – 20 = 80°C \)

So,

\(\displaystyle Q = 500 \times 0.385 \times 80 \)

Q = 15400 J

The heat required is 15400 J.

Problem 2: How much energy is needed to raise the temperature of 2 kg of water from 25°C to 75°C? The specific heat capacity of water is 4184 J/kg°C.

Solution:

• Mass (m) = 2 kg
• Initial temperature (T_i) = 25°C
• Final temperature (T_f) = 75°C
• Specific heat capacity of water (c) = 4184 J/kg°C

The heat required (Q) is given by:

\(\displaystyle Q = mc\Delta T \)

where (\(\displaystyle \Delta T = T_f – T_i \)):

\(\displaystyle \Delta T = 75 – 25 = 50°C \)

So,

\(\displaystyle Q = 2 \times 4184 \times 50 \)

Q = 418400 J

The energy needed is 418400 J.

Problem 3: Calculate the amount of heat required to raise the temperature of 1 mole of oxygen gas (O2) from 20°C to 70°C at constant volume. The molar specific heat capacity at constant volume for oxygen is 21 J/mol°C.

Solution:

• Number of moles (n) = 1 mol
• Initial temperature (T_i) = 20°C
• Final temperature (T_f) = 70°C
• Molar specific heat capacity at constant volume (C_v) = 21 J/mol°C

The heat required (Q) is given by:

\(\displaystyle Q = nC_v\Delta T \)

where (\(\displaystyle \Delta T = T_f – T_i \)):

\(\displaystyle \Delta T = 70 – 20 = 50°C \)

So,

\(\displaystyle Q = 1 \times 21 \times 50 \)

Q = 1050 J

The heat required is 1050 J.

Problem 4: How much heat is required to raise the temperature of 2 moles of nitrogen gas (N₂) from 15°C to 45°C at constant pressure? The molar specific heat capacity at constant nitrogen pressure is 29 J/mol°C.

Solution:

• Number of moles (n) = 2 mol
• Initial temperature (T_i) = 15°C
• Final temperature (T_f) = 45°C
• Molar specific heat capacity at constant pressure (C_p) = 29 J/mol°C

The heat required (Q) is given by:

\(\displaystyle Q = nC_p\Delta T \)

where (\(\displaystyle \Delta T = T_f – T_i \)):

\(\displaystyle \Delta T = 45 – 15 = 30°C \)

So,

\(\displaystyle Q = 2 \times 29 \times 30 \)

Q = 1740 J

The heat required is 1740 J.

Problem 5: Compare the heat required to raise the temperature of 1 kg of aluminum and 1 kg of iron from 25°C to 75°C. The specific heat capacities are 900 J/kg°C for aluminum and 450 J/kg°C for iron.

Solution:

• Mass (m) = 1 kg
• Initial temperature (T_i) = 25°C
• Final temperature (T_f) = 75°C
• Specific heat capacity of aluminum (c_Al) = 900 J/kg°C
• Specific heat capacity of iron (c_Fe) = 450 J/kg°C

For aluminum:

\(\displaystyle Q_{Al} = mc_{Al}\Delta T \)

\(\displaystyle \Delta T = 75 – 25 = 50°C \)

\(\displaystyle Q_{Al} = 1 \times 900 \times 50 \)

\(\displaystyle Q_{Al} = 45000 \, J \)

For iron:

\(\displaystyle Q_{Fe} = mc_{Fe}\Delta T \)

\(\displaystyle \Delta T = 75 – 25 = 50°C \)

\(\displaystyle Q_{Fe} = 1 \times 450 \times 50 \)

\(\displaystyle Q_{Fe} = 22500 \, J \)

The heat required to raise the temperature of 1 kg of aluminum is 45000 J, and for 1 kg of iron, it is 22500 J.

Problem 6: Calculate the heat required to raise the temperature of 3 moles of argon gas (Ar) from 300 K to 600 K at constant volume. The molar specific heat capacity at constant volume for argon is 12.5 J/mol·K.

Solution:

• Number of moles (n) = 3 mol
• Initial temperature (T_i) = 300 K
• Final temperature (T_f) = 600 K
• Molar specific heat capacity at constant volume (C_v) = 12.5 J/mol·K

The heat required (Q) is given by:

\(\displaystyle Q = nC_v\Delta T \)

where (\(\displaystyle\Delta T = T_f – T_i \)):

\(\displaystyle\Delta T = 600 – 300 = 300 \, K \)

So,

\(\displaystyle Q = 3 \times 12.5 \times 300 \)

Q = 11250 J

The heat required is 11250 J.

FAQs