The story of optical instruments is as old as civilization itself, beginning with the ancient Egyptians and Mesopotamians who first developed simple lenses. These early lenses were often made from polished crystal or glass and were primarily used to start fires or for magnification.
As we move forward in time, the ancient Greeks made significant contributions to optics. The philosopher Empedocles suggested that vision works with light rays entering the eye, a theory that would be refined by others such as Euclid and Ptolemy. Euclid, in particular, wrote extensively on optics, focusing on the geometry of light and vision.
The Islamic Golden Age saw a flourishing of science and knowledge, with scholars like Alhazen (Ibn al-Haytham) making groundbreaking advances in optics. Alhazen’s work on the camera obscura and his book, “Book of Optics,” laid the foundation for the modern understanding of light and vision.
Optics continued to develop in medieval Europe, with spectacles being invented in Italy in the 13th century. These early glasses were simple reading aids with convex lenses to help the aging eyes of scholars and monks.
The Renaissance period was a time of great advancement in optical instruments. Johannes Kepler and Galileo Galilei improved the design of telescopes, allowing humans to look at the heavens in ways never before possible. Galileo’s use of the telescope to observe the moons of Jupiter revolutionized astronomy and further cemented the importance of optical instruments in scientific discovery.
The 17th century saw further developments with the invention of the microscope in the Netherlands. This allowed scientists to explore the world of the very small, leading to discoveries in biology and medicine.
In the centuries that followed, optical instruments became more sophisticated with the development of different lenses and mirrors. The understanding of light also evolved, with scientists like Isaac Newton studying the dispersion of light and the nature of colors.
Today, optical instruments are ubiquitous and essential in various fields, from medicine to communication technology. They have allowed us to explore the universe, understand the microscopic world, and improve our quality of life through vision correction.
What are Optical Instruments?
Optical instruments are devices that manipulate light to enhance, magnify, or change the appearance of objects. They include a wide range of tools from glasses to complex scientific equipment. Optical instruments are tools we use to control light to achieve a particular result, usually to make something appear larger, clearer, or otherwise different from how it would look to the naked eye. They work by bending, reflecting, or refracting light waves to alter how we perceive visual information.
At their core, all-optical instruments rely on the fundamental principles of optics—the branch of physics that studies the behavior and properties of light. This includes how light interacts with matter, how it behaves when it encounters different materials, and how it can be harnessed to improve our vision or help us see things that are far away, very small, or otherwise beyond the capabilities of our eyes alone.
In essence, optical instruments enhance our ability to see and analyze the world around us, extending the reach of our natural vision. They are a testament to human ingenuity, allowing us to explore everything from the vastness of space to the intricacies of cells.
Different Types of Optical Instruments
There are several types of optical instruments, each serving different purposes. Common examples include microscopes, telescopes, cameras, and spectacles.
Eye: The eye is a natural optical instrument that allows us to see. It works similarly to a camera, with a lens system that focuses light onto a light-sensitive surface called the retina. The retina converts the light into electrical signals sent to the brain to create the images we see.
Microscope: A microscope is an instrument that magnifies tiny objects, making them visible to the human eye. It uses a combination of lenses to achieve high magnification. The objective lens provides the initial magnification, which is then increased by the eyepiece lens.
Telescope: A telescope is designed to observe distant objects by collecting and focusing light. There are two main types:
- Refracting telescopes use lenses to bend light and bring it to a focus.
- Reflecting telescopes use mirrors to gather and focus light.
Both types allow us to see faraway stars, planets, and galaxies that are otherwise invisible to the naked eye.
Kaleidoscope: A kaleidoscope is an optical instrument that creates beautiful patterns through multiple reflections. It consists of two or more mirrors positioned at an angle to each other. When light enters the kaleidoscope, it reflects off the mirrors and produces symmetrical patterns from the objects inside.
Periscope: A periscope allows you to see over, around, or through an obstacle. It uses a set of mirrors or prisms at each end of a tube to reflect images from one end to the other. Periscopes are commonly used in submarines to allow viewing above the water’s surface while the submarine remains submerged.
These instruments are all based on the principles of optics and have been developed to extend our natural vision, each serving a unique purpose and allowing us to explore and understand the world in different ways.
The Eye
The human eye is a natural optical instrument that captures light and processes it to form images. When we talk about optical instruments, it’s fascinating to consider that one of the most sophisticated ones is a part of us—the human eye. To understand the eye as an optical instrument, let’s think about what an optical instrument does: it processes light to create images.
The eye, in its essence, is an incredibly efficient optical system. It’s designed to take in light and convert it into signals that our brain can interpret as visual images. It does this through a series of steps that involve bending (refracting) light and focusing it to form a clear picture.
Here’s a simplified way to look at it: imagine the eye as a camera. A camera has a lens that focuses light onto a sensor to create an image. Similarly, the eye has structures that focus light onto the retina, which acts like the sensor in a camera. The retina then sends signals to the brain, which processes them into the images we see.
The eye’s ability to adjust to different lighting conditions and focus on objects at various distances is akin to a camera adjusting its aperture and lens focus. This adaptability is what makes the eye such a remarkable and complex optical instrument. The human eye is a remarkable organ that allows us to perceive the world around us. Let’s break down how it works and understand some common vision defects using the provided images.
Structure and Function of the Eye: The figure illustrates the structure of the eye:
- Cornea: Light first enters the eye through the cornea, which is the curved front surface.
- Pupil and Iris: The light then passes through the pupil, the central hole in the iris. The size of the pupil can change to control the amount of light entering the eye.
- Lens: After passing through the pupil, light is focused by the eye’s crystalline lens onto the retina.
- Retina: The retina is a layer of nerve fibers at the back of the eye. It contains rods and cones, which detect light intensity and color, respectively. These cells send electrical signals to the brain via the optic nerve, allowing us to process visual information.
- Ciliary Muscles: The shape and focal length of the lens can be adjusted by the ciliary muscles to focus on objects at different distances.
Accommodation
When the ciliary muscles are relaxed, the focal length of the lens is about 2.5 cm, which focuses distant objects sharply on the retina. When an object is closer, the ciliary muscles contract to shorten the focal length of the lens, maintaining a sharp image on the retina. This ability of the eye to adjust its focus is called accommodation. The closest distance at which the eye can focus on an object is known as the near point, typically about 25 cm for a young adult. This distance increases with age due to reduced muscle effectiveness and lens flexibility, a condition called presbyopia.
Common Vision Defects:
Nearsightedness (Myopia)
Nearsightedness, also known as myopia, is a common vision condition where people can see nearby objects clearly, but objects farther away appear blurry.

Myopia occurs when the eyeball is too long relative to the focusing power of the cornea and lens of the eye. This causes light rays to focus at a point in front of the retina, rather than directly on its surface. Because the light focuses in front of the retina, distant objects don’t appear sharp. The further away an object is, the blurrier it will look to someone with myopia.
Myopia can be due to the shape of the eye itself, where the eyeball grows too long from front to back. It can also be because the cornea, the clear front cover of the eye, is too curved for the length of the eyeball. In some cases, the lens inside the eye is too thick.
Myopia is typically corrected with glasses or contact lenses, which help to redirect the light rays to focus on the retina, allowing for clear vision at a distance. This is corrected with a concave lens, which diverges the light rays so they focus on the retina. Refractive surgery is another option for correcting myopia, where the shape of the cornea is changed permanently.
Farsightedness (Hypermetropia):
Farsightedness, known scientifically as hypermetropia, is a common vision defect where distant objects can be seen clearly, but close ones appear blurry. Hypermetropia occurs when the eyeball is shorter than normal or the cornea (the clear front cover of the eye) has too little curvature. As a result, light entering the eye focuses behind the retina instead of on it.
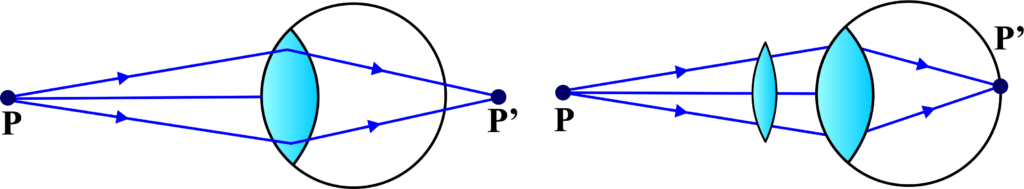
Because the light focuses behind the retina, the images of close objects are not formed correctly, leading to blurred vision when looking at things like books or screens. The condition can arise from the natural shape of the eye or develop as the eye changes with age. It can also be influenced by genetic factors.
This condition is corrected with a convex lens, which converges the light rays to focus on the retina. Glasses or contact lenses with convex lenses are used to correct hypermetropia. These lenses help converge the light rays so they focus directly on the retina, allowing for clear vision up close.
Astigmatism:
Astigmatism is a type of refractive error in the eye that causes blurred vision. It occurs when the cornea (the clear front layer of the eye) or the lens inside the eye has an irregular shape. Instead of being perfectly spherical like a baseball, the cornea or lens is shaped more like a football or the back of a spoon. This irregular shape prevents light from focusing properly on the retina, the light-sensitive surface at the back of the eye.
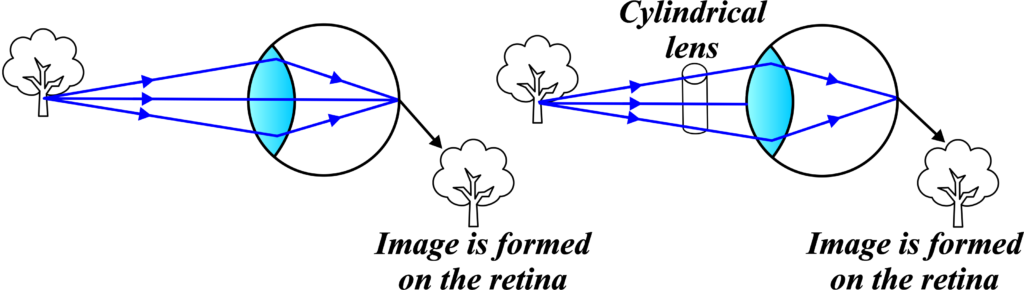
In a normal eye, the cornea and lens have a smooth curvature that bends (refracts) incoming light to meet at a single point on the retina, resulting in a clear image. However, in an eye with astigmatism, the uneven curvature causes light to refract more in one direction than the other, leading to multiple focus points, either in front of or behind the retina, or both.
This results in a distortion of vision at all distances. Common symptoms of astigmatism include blurry vision, eye strain, headaches, and difficulty with night vision. The severity of astigmatism can range from mild to extreme, and it can be present at birth or develop later in life.
Astigmatism is commonly corrected with glasses or contact lenses that have a special cylindrical lens prescription to compensate for the irregular shape of the cornea or lens. In some cases, refractive surgery may be considered.
Our eyes are incredibly important, allowing us to experience the world visually. It’s crucial to take good care of them and protect them from potential harm. Even with proper care, vision defects can occur, but most can be corrected with lenses. For those who face vision challenges, their resilience and ability to adapt are truly commendable.
Parts of the Eye:
When we discuss optical instruments, it’s essential to understand the parts of the human eye, as it is one of the most complex and naturally occurring optical systems.
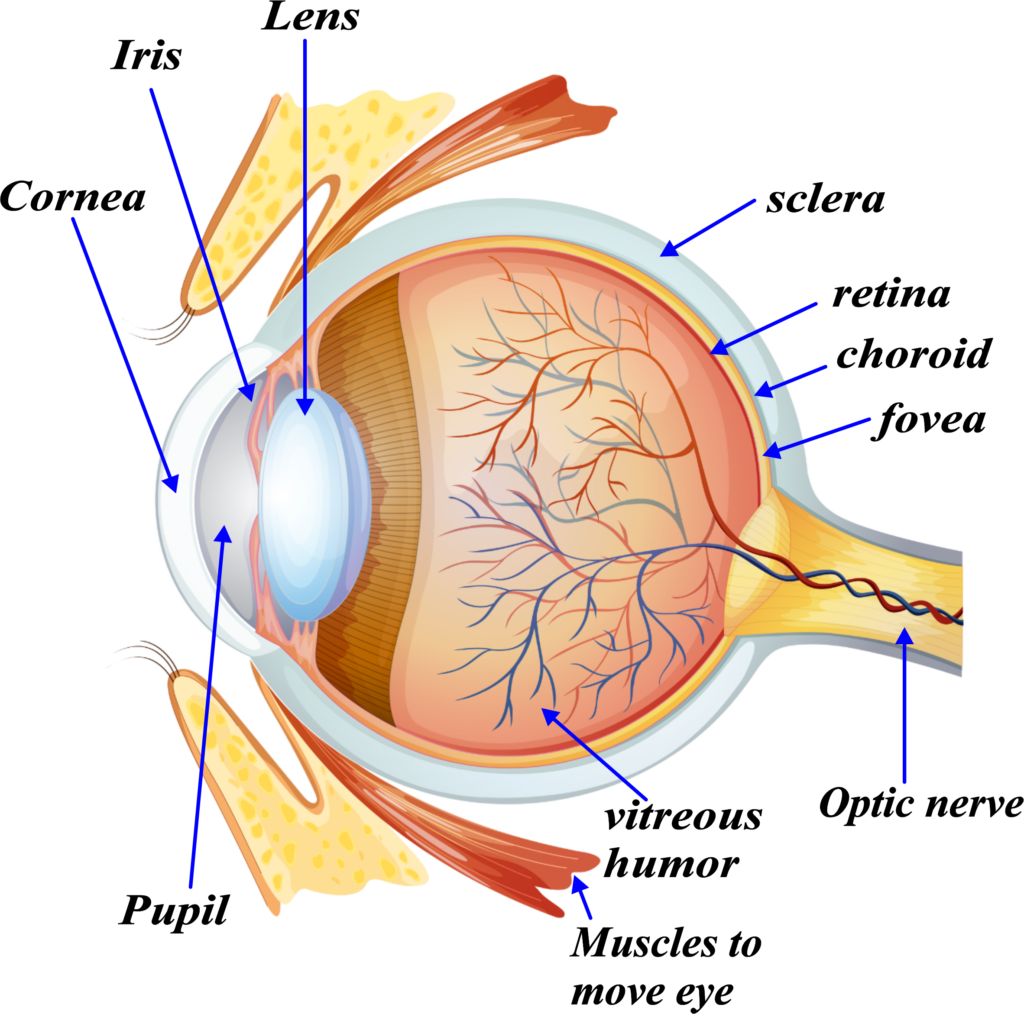
Cornea: The cornea is the clear, dome-shaped surface that covers the front of the eye. It acts as the eye’s primary lens, bending incoming light to help focus it onto the retina.
Iris: The iris is the colored part of the eye surrounding the pupil. It controls the size of the pupil and, consequently, the amount of light that enters the eye.
Pupil: The pupil is the black circular opening in the iris that lets light in. Its size changes in response to light intensity.
Lens: The lens is a clear, flexible structure that works with the cornea to focus light correctly on the retina. It changes shape to adjust the eye’s focus, allowing us to see objects at different distances.
Retina: The retina is the light-sensitive layer of tissue at the back of the inner eye. It acts like the film in a camera, capturing the image formed by the cornea and lens.
Optic Nerve: The optic nerve is the cable that carries the visual information from the retina to the brain, where it is interpreted as the images we see.
Sclera: The sclera is the white part of the eye, a tough covering that protects the eyeball.
Conjunctiva: The conjunctiva is a thin, clear membrane that covers the sclera and lines the inside of the eyelids. It helps keep the front surface of the eye moist and protected.
Aqueous Humor: Aqueous humor is a clear fluid that fills the space between the cornea and the iris, helping to maintain the shape of the eye and providing nutrients to the eye’s internal structures.
Vitreous Humor: The vitreous humor is a clear, jelly-like substance that fills the large space in the middle of the eye, helping to keep the retina in place and maintain the eye’s shape.
Working of the Eye:
To understand the workings of the eye in the context of optical instruments, let’s consider the eye as a system that captures and processes light to create visual images. Light enters the eye through the cornea, passes through the pupil, is focused by the lens, and forms an image on the retina. This image is then transmitted to the brain via the optic nerve.
- Light Entry: It all starts when light enters the eye through the cornea, the clear front layer that acts like a camera lens, directing the light inward.
- Iris and Pupil: The light then passes through the pupil, the black opening in the center of the iris, which is the colored part of the eye. The iris adjusts the size of the pupil to control how much light enters, similar to the aperture on a camera.
- Lens Adjustment: Behind the pupil is the lens, which fine-tunes the focus of the light. The lens can change shape, thanks to the surrounding ciliary muscles, to focus on objects at different distances—a process known as accommodation.
- Retina – The Image Sensor: The focused light reaches the retina, a layer of light-sensitive cells at the back of the eye. The retina works like the film in a camera or the sensor in a digital camera. It contains two types of cells: rods, which are sensitive to light and help us see in low-light conditions, and cones, which detect color.
- Image Processing: The rods and cones convert the light into electrical signals. These signals are then sent through the optic nerve to the brain.
- Brain Interpretation: The brain receives the signals and interprets them, allowing us to perceive the shapes, colors, and movements of the world around us. Interestingly, the images projected on the retina are upside down, but our brain flips them right side up.
This process happens continuously and rapidly, giving us a seamless experience of sight.
Microscope
A microscope is a precision instrument used to see objects that are too small for the naked eye. The basic idea of a microscope is to use lenses to magnify an image, allowing us to observe details that would otherwise be impossible to detect.
Microscopes have been instrumental in numerous scientific discoveries and continue to be a vital tool in many fields, including biology, medicine, and materials science. They help us to explore the microscopic world, from examining cell structures to identifying bacteria and viruses.
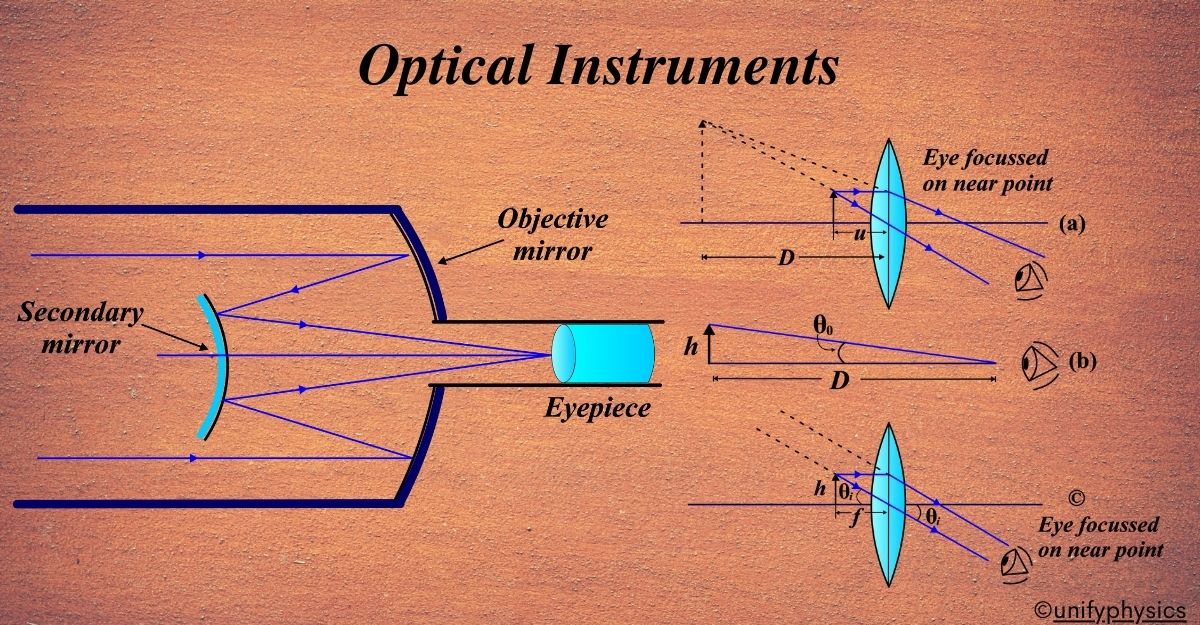
A simple magnifier, or microscope, is essentially a converging lens with a short focal length. When using this lens as a microscope, you position it near the object, typically at or closer than its focal length, and place your eye on the other side of the lens. The goal is to get an enlarged, upright, and virtual image of the object at a distance that can be comfortably viewed, ideally at 25 cm or more.
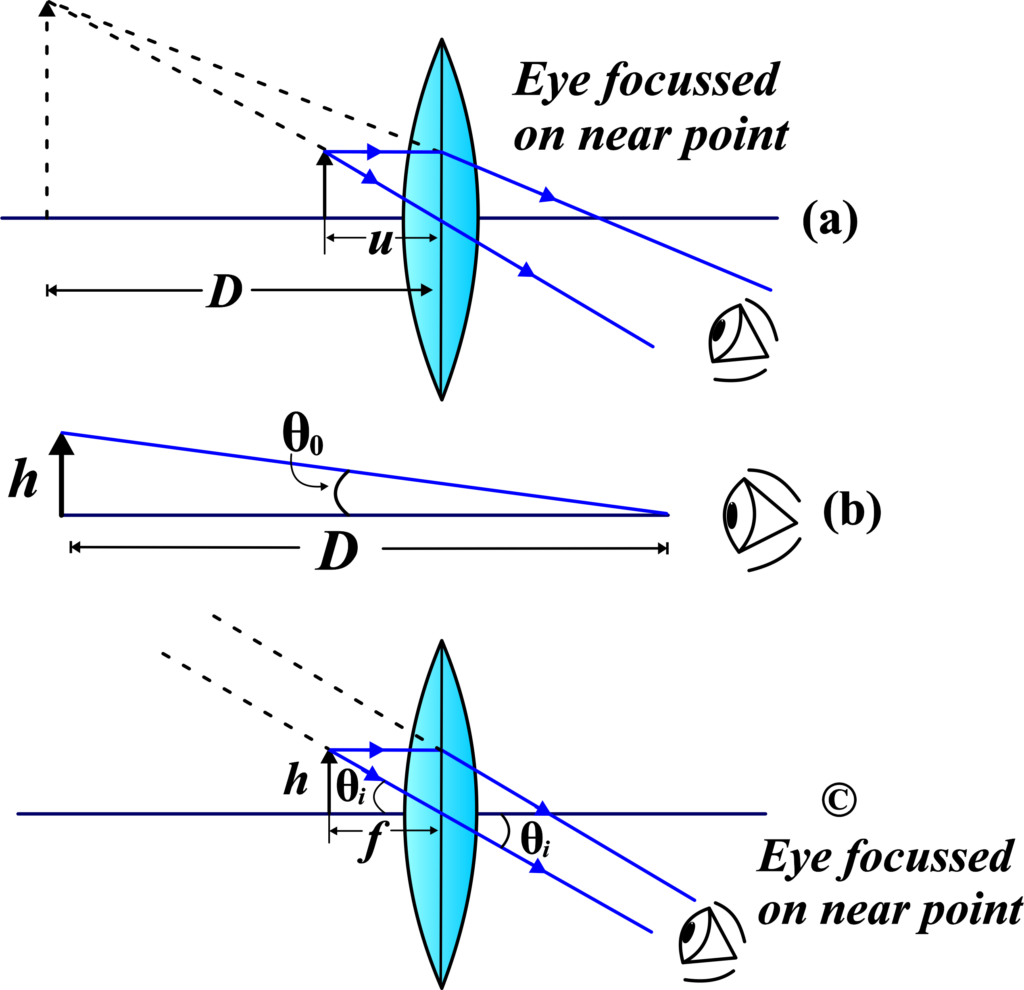
If the object is exactly one focal length away, the image forms at infinity, making it easy to view for a relaxed eye. If the object is slightly less than one focal length away, the image appears closer than infinity. Though the most comfortable viewing distance is at the near point (around 25 cm), this can strain the eye. Hence, viewing at infinity is often preferred. This is illustrated as shown in Fig.
To calculate the linear magnification (m) for an image formed at the near point (D), use the equation:
\(\displaystyle m = \frac{v}{u} = v \left( \frac{1}{v} – \frac{1}{f} \right) = \left( 1 – \frac{v}{f} \right) \)
With (v) being negative and equal in magnitude to (D), the magnification becomes:
\(\displaystyle m = \left( 1 + \frac{D}{f} \right) \)
Given (\(\displaystyle D \approx 25\) ) cm, to achieve a magnification of six, you need a convex lens with a focal length (f = 5) cm. Here, ( \(\displaystyle m = \frac{h’}{h} \)), where (h) is the object’s size and (h’) is the image’s size. This also equals the ratio of the angle subtended by the image to the angle subtended by the object when placed at (D) for comfortable viewing.
In the case of viewing at infinity, we calculate the angular magnification. Suppose the object height is (h). The maximum angle (\(\displaystyle \theta_0\) ) it can subtend without a lens when at the near point (D), is:
\(\displaystyle \tan \theta_0 \approx \theta_0 = \frac{h}{D} \)
Using the lens, if the object is at (u), the angle (\(\displaystyle \theta_i \)) subtended by the image is:
\(\displaystyle \tan \theta_i \approx \theta_i = \frac{h}{u} \)
When the object is at ( u = -f ):
\(\displaystyle \theta_i = \frac{h}{f} \)
Therefore, the angular magnification is:
\(\displaystyle m = \frac{\theta_i}{\theta_0} = \frac{D}{f} \)
This is one less than the magnification when the image is at the near point but more comfortable for viewing, and the difference in magnification is usually minor.
When discussing optical instruments like microscopes and telescopes, we typically assume the image forms at infinity. A simple microscope can achieve a maximum magnification of about 9 for practical focal lengths.
Parts of Microscope:
Typical parts of a microscope include the eyepiece, objective lenses, stage, and illumination source. Here’s a breakdown of the main components:
Eyepiece (Ocular Lens): The eyepiece is the lens that you look through at the top of the microscope. It usually contains a 10x or 15x power lens that magnifies the image of the specimen.
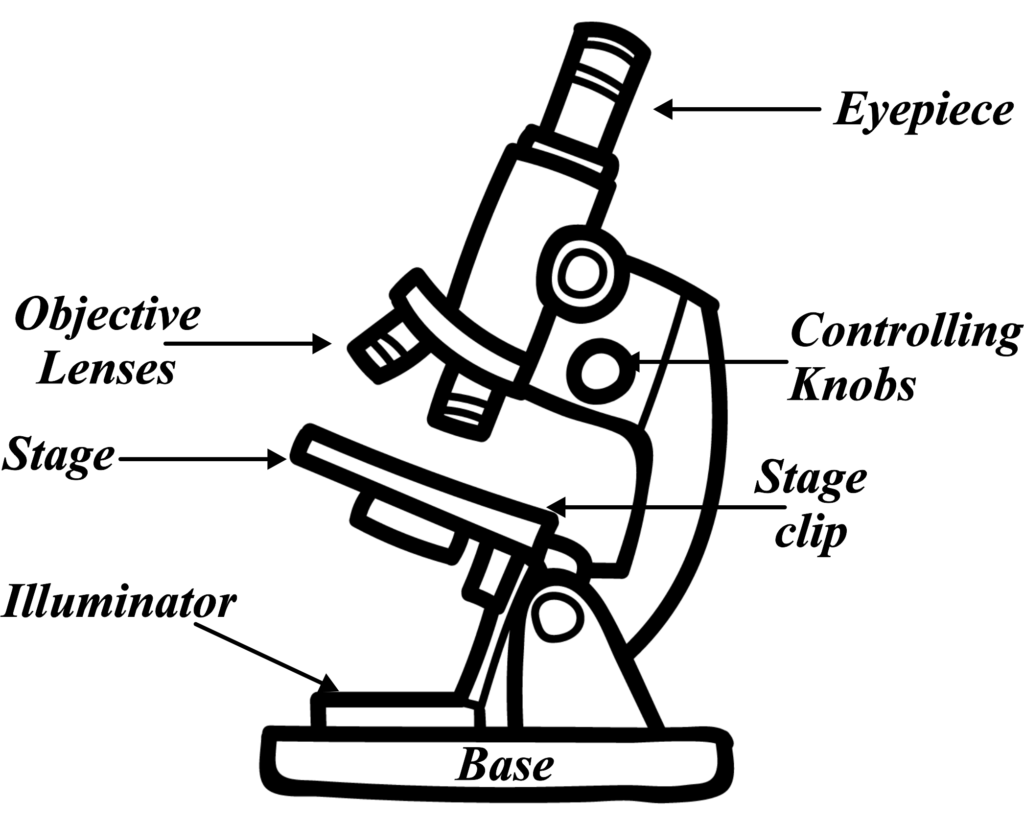
Objective Lenses: These are the primary optical lenses on a microscope, and they are located on a rotating nosepiece just above the stage. They range in power from 4x to 100x. When paired with the eyepiece, these lenses further magnify the image of the specimen.
Stage: The stage is the flat platform where the slides are placed for observation. It has a hole in the center to allow light to pass through both the stage and the specimen.
Stage Clips: Stage clips hold the slides in place. If your microscope has a mechanical stage, you will be able to move the slide around by turning two knobs. One moves it left and right, and the other moves it up and down.
Condenser: The condenser is a lens designed to focus the light onto the specimen. It is generally found under the stage and its position can be adjusted.
Illuminator: The illuminator is the light source for the microscope, usually situated under the stage. It shines light upwards through the specimen to allow you to see it.
Diaphragm or Iris: The diaphragm or iris controls the amount of light that reaches the specimen. It is located above the condenser and below the stage.
Controlling Knobs: There are typically two sets of controlling knobs. The coarse adjustment knob moves the stage up and down to help you get the specimen into view. The fine adjustment knob is used to fine-tune the focus on the specimen once it is in view. These components work together to allow the microscope to function properly and are essential for viewing specimens in detail.
Working of Microscope
Microscopes magnify small objects by using lenses to focus light, creating an enlarged image for detailed observation.
- Light Source: At the base of the microscope, there’s a light source that illuminates the specimen. This light is crucial because it helps to make the specimen visible through the microscope.
- Condenser Lens: Above the light source, the condenser lens focuses the light onto the specimen. It doesn’t magnify the image but ensures that the light is directed properly to get a clear view.
- Specimen on Stage: The specimen is placed on the stage, which is the flat platform of the microscope. It’s held in place by clips and positioned so that the light shines through it.
- Objective Lens: Above the stage, the objective lens collects light from the specimen. This lens is responsible for the primary magnification of the specimen. It creates a real, inverted image of the specimen inside the microscope.
- Eyepiece or Ocular Lens: The real image created by the objective lens is then magnified further by the eyepiece. This lens is what you look through to see the magnified image.
- Total Magnification: The total magnification of the microscope is the product of the magnifications of the objective lens and the eyepiece. For example, if the objective lens magnifies 40x and the eyepiece magnifies 10x, the total magnification would be 400x.
- Focusing Knobs: Microscopes have knobs that adjust the focus. The coarse adjustment knob moves the stage up and down to bring the specimen into the general focus, while the fine adjustment knob makes smaller adjustments for a clearer image.
A microscope works by directing light through a series of lenses to magnify a small object, allowing us to see details that would otherwise be invisible. It’s a brilliant application of the principles of light and magnification that enables us to explore the microscopic world.
Types of Microscopes: Microscopes come in various types, such as compound, stereo, and electron microscopes.
Simple Microscope:
A simple microscope is essentially a magnifying glass that uses a single convex lens to enlarge the image of an object. It’s the most basic form of microscope, often used for low magnification.
- Convex Lens: The key component of a simple microscope is a convex lens, which is thicker at the center than at the edges. This shape causes light rays coming from the object being viewed to bend inwards (converge).
- Magnification: When an object is placed close to the lens, within its focal length, the light rays after passing through the lens diverge. The eye then perceives these diverging rays as coming from a much larger object, thus creating a magnified image.
- Virtual Image: The image formed by a simple microscope is virtual, meaning it cannot be projected on a screen. It’s seen when looking through the lens and appears erect and larger than the actual object.
- Magnifying Power: The magnifying power of a simple microscope can be calculated using the formula:
\(\displaystyle M = 1 + \frac{D}{f} \)
where (M) is the magnification, (D) is the least distance of distinct vision (usually taken as 25 cm), and (f) is the focal length of the convex lens.
A simple microscope enlarges the appearance of an object by bending light in such a way that the eye perceives a larger image.
Compound Microscope
A compound microscope has more than one lens. It typically has an objective lens close to the object being viewed and an eyepiece lens through which the viewer looks. The combination of lenses provides a higher level of magnification and detail.
- Objective Lens: This is the primary lens that is closest to the specimen. It has a short focal length and is responsible for creating an enlarged image of the object being observed. This image is known as the real image because it can be projected onto a screen or a layer of photographic film.
- Eyepiece (Ocular Lens): The real image created by the objective lens is then magnified further by the eyepiece. This lens is closer to the observer’s eye and enlarges the real image to create a much larger virtual image, which is what the observer sees.
- Total Magnification: The total magnification of a compound microscope is the product of the magnifications of the objective and the eyepiece lenses. For instance, if the objective lens magnifies 40x and the eyepiece lens magnifies 10x, the total magnification would be 400x.
- Light Path: In a compound microscope, light from an illuminator passes through the specimen. The objective lens captures the light from the specimen and magnifies it to create the real image. This image is then magnified again by the eyepiece, allowing the observer to see a much larger version of the specimen.
- Focus Adjustment: Compound microscopes have knobs for adjusting the focus. The coarse adjustment knob brings the specimen into the general focus, and the fine adjustment knob is used for precise focusing to get a clear image.
The compound microscope is a powerful tool in science that allows us to see and study objects at a cellular or even molecular level by significantly magnifying them through a series of lenses.
Electron Microscope: An electron microscope uses a beam of electrons instead of light to create an image. It has much higher magnification and resolving power than a light microscope, allowing it to see much smaller objects in finer detail.
Stereo Microscope: A stereo microscope, also known as a dissecting microscope, provides a three-dimensional view of the specimen. It’s used for viewing larger, solid specimens at lower magnification.
Scanning Probe Microscope: A scanning probe microscope measures the surface of a specimen using a physical probe. It can create images of surfaces at the atomic level.
Magnification in Microscope
In the context of optical instruments, specifically microscopes, magnification refers to the process of enlarging the appearance of an object. Magnification in a microscope is achieved by the combination of the eyepiece and objective lenses, each contributing to the overall enlargement of the image. Magnification is the process of enlarging the appearance of an object through an optical instrument. In the case of a microscope, it’s how much larger the microscope makes the object appear compared to its actual size.
A microscope uses lenses to magnify objects. There are two main lenses involved:
- Objective Lens: This lens is close to the object and has a high magnification power.
- Eyepiece Lens: Also known as the ocular lens, this is the lens you look through, and it further magnifies the image formed by the objective lens.
The total magnification of a microscope is calculated by multiplying the magnification power of the objective lens by the magnification power of the eyepiece lens. For example, if the objective lens magnifies 40x and the eyepiece lens magnifies 10x, the total magnification would be ( 40 × 10 = 400x ).
The formula for magnification is given by:
\(\displaystyle M = 1 + \frac{D}{f} \)
- (M) is the magnification,
- (D) is the least distance of distinct vision (usually taken as 25 cm for the human eye),
- (f) is the focal length of the lens.
This formula tells us that magnification depends on the focal length of the lens. A shorter focal length means a higher magnification because the lens can bend light rays more sharply, bringing them to focus closer to the lens and creating a larger image.
Types of Magnification in Microscopes:
Angular Magnification: This is the magnification that occurs when an object is viewed through a lens, such as the eyepiece of a microscope. The formula for angular magnification (M) is:
\(\displaystyle M = 1 + \frac{D}{f} \)
- (D) is the least distance of distinct vision (typically around 25 cm for the human eye),
- (f) is the focal length of the lens.
Linear Magnification: This type of magnification refers to the ratio of the size of the image seen through the microscope to the actual size of the object. In a compound microscope, linear magnification is the product of the magnifications provided by the objective and the eyepiece lenses.
The formula for total magnification (Mtotal) in a compound microscope is:
\(\displaystyle M_{total} = M_{objective} \times M_{eyepiece} \)
- (Mobjective) is the magnification of the objective lens,
- (Meyepiece) is the magnification of the eyepiece lens.
It’s important to note that while magnification makes objects appear larger, it does not improve the resolution, which is the ability to distinguish between two separate points. Higher magnification without adequate resolution may just result in a larger but blurrier image.
Angular Magnification Due to the Eyepiece: The eyepiece lens further magnifies the image produced by the objective lens. Its angular magnification (Mep) is also given by:
\(\displaystyle M_{ep} = 1 + \frac{D}{f_{ep}} \)
where (fep) is the focal length of the eyepiece.
Magnification When the Image is Formed at Infinity: When a compound microscope is adjusted for the final image to be at infinity, the total magnification is given by:
\(\displaystyle M_{total} = \frac{D}{f_{obj}} \times \frac{N}{f_{ep}} \)
- (N) is the near point distance of the eye (usually 25 cm),
- (fobj) is the focal length of the objective lens,
- (fep) is the focal length of the eyepiece.
This configuration allows for more relaxed viewing and is often used in microscopes designed for prolonged use, such as those in research labs.
Telescope
A telescope is an optical instrument that significantly enlarges distant objects, making them more visible to the observer. It’s a fundamental tool in astronomy and has been instrumental in many scientific discoveries.
- Light Gathering: The primary purpose of a telescope is to collect as much light as possible from a distant object. The more light that’s gathered, the brighter and clearer the image will be. This is particularly important for viewing dim objects in the night sky, such as stars and galaxies.
- Magnification: Once light is gathered, a telescope uses lenses or mirrors to magnify the image. Magnification makes the object appear larger and more detailed, allowing us to observe features that would otherwise be too small to see with the naked eye.
Telescopes can be quite large, especially those used in professional astronomy, because a larger size means more light can be collected, resulting in better images. However, even smaller telescopes used by hobbyists can provide impressive views of the moon, planets, and stars.
Telescopes extend our vision far beyond the capabilities of our eyes, enabling us to explore the universe from our own backyards. They are a perfect example of how optical principles can be applied to enhance our understanding of the cosmos.
Parts of Telescope:
Telescopes consist of an objective lens or mirror, an eyepiece, and often a mount and finderscope.
Objective Lens or Primary Mirror: The objective lens (in refracting telescopes) or primary mirror (in reflecting telescopes) is the most important part of a telescope. It’s the first element that encounters light from distant objects. Its main function is to gather light and create an image inside the telescope.
Eyepiece: The eyepiece is a smaller lens located at the viewing end of the telescope. It magnifies the image produced by the objective lens or primary mirror, allowing us to see a larger version of the distant object.
Tube: The tube holds the optical components of the telescope in alignment. It protects the inside parts from dust, moisture, and light that could interfere with the image.
Mount: The mount supports the telescope and allows it to move and point to different parts of the sky. There are two main types of mounts: altazimuth and equatorial. The altazimuth mount moves up, down, left, and right, while the equatorial mount is aligned with the Earth’s axis and can follow the rotation of the sky.
Focuser: The focuser is a mechanism that holds the eyepiece and allows for precise adjustments to bring the image into sharp focus. It usually consists of a set of gears that move the eyepiece in and out along the telescope’s axis.
Finderscope: The finderscope is a small, low-power telescope mounted on the side of the main telescope. It has a wider field of view and helps in locating objects to observe with the main telescope.
Diagonal: In some telescopes, especially refractors, there is a diagonal mirror or prism that bends the light path, usually at a 90-degree angle, to make viewing more comfortable. It’s placed between the eyepiece and the objective lens or primary mirror.
Working of Telescope:
Telescopes gather light from distant objects and focus it to create a magnified image for observation.
- Light Collection: The main job of a telescope is to collect light. It does this with a large lens or mirror called the objective. This component is crucial because the more light the telescope can collect, the better we can see distant objects.
- Focusing: After light is collected, it needs to be brought to focus. In refracting telescopes, this is done by the objective lens, which bends the light to a focal point. In reflecting telescopes, a curved mirror gathers the light and reflects it to a focus.
- Magnification: Once the light is focused, it passes through the eyepiece, which is a smaller lens that magnifies the image. The eyepiece works like a magnifying glass, enlarging the focused light so that when you look through it, the object appears bigger.
- Image Formation: The combination of the objective and the eyepiece creates an enlarged image of the distant object, allowing us to see details that are not visible to the naked eye. The final image can be upside down or reversed, depending on the design of the telescope.
Telescopes work by collecting a lot of light from a distant object and then using lenses or mirrors to focus and magnify that light, creating an image that is much larger and clearer than what the human eye could see on its own. This process enables us to observe the wonders of the night sky in great detail.
Types of Telescope
There are refracting, reflecting, and catadioptric telescopes, each using different methods to collect and focus light.
Refracting Telescopes: Refracting telescopes, or refractors, use lenses to bend (refract) light to a focus. They consist of an objective lens, which is the main lens at the front of the telescope, and an eyepiece at the back. The objective lens gathers light and focuses it to create an image, which is then magnified by the eyepiece.
A telescope is a device that magnifies far-off objects. It consists of two main parts: the objective and the eyepiece. The objective has a longer focal length and a larger aperture compared to the eyepiece.
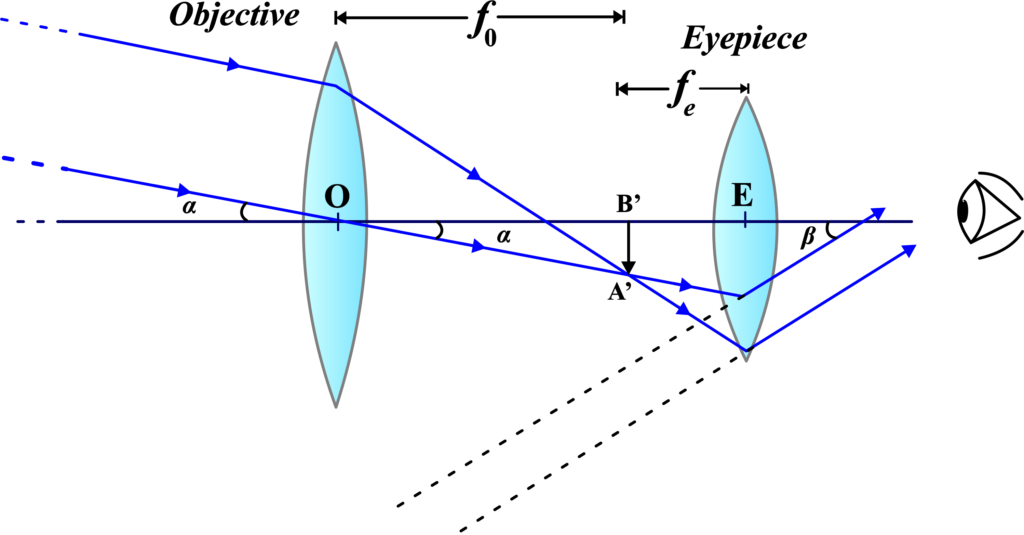
When light from a distant object enters the telescope, it first hits the objective. This forms a real image at the second focal point within the telescope tube. The eyepiece then magnifies this real image, resulting in a larger, upside-down final image.
The magnifying power, denoted as ‘m’, is calculated as the ratio of the angle (β) subtended at the eye by the final image to the angle (α) subtended at the lens or the eye by the object. Mathematically, it can be approximated as:
\(\displaystyle m = \frac{\beta}{\alpha} \approx \frac{f_o}{f_e} \)
Here, (fo) is the focal length of the objective and (fe) is the focal length of the eyepiece. The length of the telescope tube is the sum of these two focal lengths.
Terrestrial telescopes have additional inverting lenses to make the final image upright. These types of telescopes are suitable for both terrestrial and astronomical observations. For instance, a telescope with an objective focal length of 100 cm and an eyepiece focal length of 1 cm would have a magnifying power of 100.
The key factors to consider in an astronomical telescope are its light-gathering power and its resolving power. The light-gathering power, which allows the observation of fainter objects, depends on the area of the objective. The resolving power, which is the ability to distinguish between two objects that are close together in the same direction, also depends on the diameter of the objective. Therefore, the goal in optical telescopes is to have a large diameter objective.
The largest lens objective currently in use has a diameter of 40 inches (~1.02 m) and is located at the Yerkes Observatory in Wisconsin, USA. However, such large lenses are heavy and challenging to manufacture and support. Moreover, it is difficult and costly to produce large lenses that form images free from chromatic aberration and distortions.
Reflecting Telescopes: Reflecting telescopes, or reflectors, use a curved mirror, known as the primary mirror, to collect light and reflect it to a focus. A secondary mirror then redirects the focused light to the eyepiece. Reflecting telescopes are popular because they can be made larger than refractors and are often less expensive to produce.
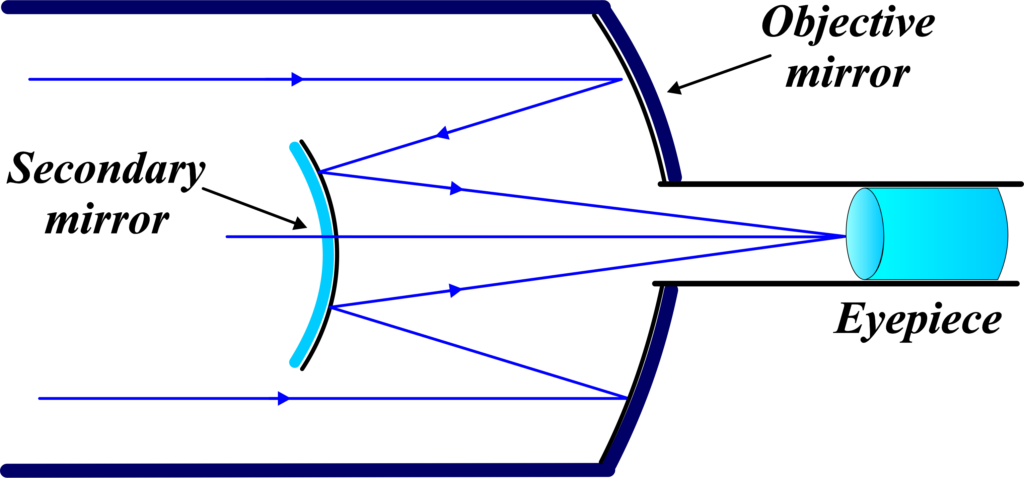
Modern telescopes prefer to use a concave mirror instead of a lens as the objective. These are known as reflecting telescopes. The advantage of using a mirror is that it doesn’t suffer from chromatic aberration, a common issue with lenses.
A mirror is also lighter than a lens of the same optical quality, making it easier to support. It can be supported across its entire back surface, not just at the edges, which simplifies the mechanical design.
However, reflecting telescopes do have a challenge. The objective mirror focuses light within the telescope tube, which can be blocked by the eyepiece or the observer. For example, in the large 200-inch (~5.08 m) diameter Mt. Palomar telescope in California, the observer is positioned near the mirror’s focal point inside a small cage.
To overcome this issue, some designs use another mirror to redirect the focused light. The Cassegrain telescope, named after its inventor, uses a convex secondary mirror to focus the incoming light, which then passes through a hole in the primary objective mirror. This design allows for a large focal length in a compact telescope.
India’s largest telescope, located in Kavalur, Tamil Nadu, is a 2.34 m diameter reflecting (Cassegrain) telescope. It was manufactured, installed, and is currently operated by the Indian Institute of Astrophysics in Bangalore. The world’s largest reflecting telescopes are the Keck telescopes in Hawaii, USA, each with a 10-meter diameter reflector.
Catadioptric Telescopes: Catadioptric telescopes combine lenses and mirrors to gather and focus light. They use a corrector plate, and a glass lens at the front of the telescope, to correct optical errors. The light is then reflected by a primary mirror to a secondary mirror, which directs it through a hole in the primary mirror to the eyepiece. This design allows for a compact telescope with a long focal length.
These are the basic types of telescopes that work on different principles of optics to bring distant objects into view. Each type has its unique features and advantages, making them suitable for various observational needs in astronomy.
Magnification in Telescope: The magnification of a telescope is determined by the focal length of the objective and the eyepiece used. Magnification is the process by which a telescope enlarges the appearance of distant objects. It’s a measure of how much larger the telescope can make something appear compared to how it looks with the naked eye.
A telescope’s magnification is achieved through the use of two lenses or mirrors: the objective lens (or mirror) and the eyepiece. The objective lens collects light from a distant object and brings it to a focus, creating an image. The eyepiece then magnifies this image.
The formula for calculating the magnification (M) of a telescope is:
\(\displaystyle M = \frac{f_{o}}{f_{e}} \)
- (fo) is the focal length of the objective lens or mirror,
- (fe) is the focal length of the eyepiece.
Example: If a telescope has an objective lens with a focal length of 1000mm and an eyepiece with a focal length of 10mm, the magnification would be: \(\displaystyle M = \frac{1000mm}{10mm} = 100x \) This means the telescope makes the object appear 100 times larger than it does to the naked eye.
Angular Magnification: When the final image is formed at infinity, which is the most comfortable viewing position for the human eye, the angular magnification (Ma) can be expressed as:
\(\displaystyle M_{a} = \frac{f_{o}}{f_{e}} \)
This is the same as the basic magnification formula and indicates how much the telescope enlarges the angle under which we see the object.
Also Read: Lens Maker’s Formula
Kaleidoscope
A kaleidoscope is a tube with mirrors that creates patterns from reflected light passing through colored objects. A kaleidoscope is an optical instrument that creates a fascinating pattern of colors and shapes, which is especially captivating to observe.
- Construction: A kaleidoscope is typically a tube containing two or more mirrors positioned at angles to each other, usually forming a triangle or a V-shape inside the tube³. At one end of the tube, there are pieces of colored glass or other transparent materials that can move freely.
- Reflection: When you look through the eyehole at the opposite end, light enters the tube and reflects off the mirrors. Because the mirrors are angled, they reflect not only the colored pieces but also each other, creating multiple reflections.
- Symmetry: The angle at which the mirrors are set causes the reflections to form a symmetrical pattern. This pattern is what you see when you look through the kaleidoscope. The symmetry comes from the fact that each mirror reflects the image of the other mirror, creating a repeating pattern.
- Variation: When you rotate the kaleidoscope, the pieces of colored glass shift and tumble, changing the pattern. Each turn creates a new, unique design because the reflections change with the position of the colored pieces.
- Principle: The principle behind a kaleidoscope is multiple reflections. The mirrors are set up so that they reflect the light multiple times before it reaches your eye. This repeated reflection is what produces complex and beautiful patterns.
A kaleidoscope uses mirrors to reflect light and colored objects in a symmetrical and ever-changing pattern, providing a simple yet profound example of the principles of reflection and symmetry in optics. It’s a delightful demonstration of how light and mirrors can work together to create artful displays of color and form.
Periscope
A periscope allows observation over, around, or through an object, obstacle, or condition that prevents direct line-of-sight observation from the viewer’s current position.
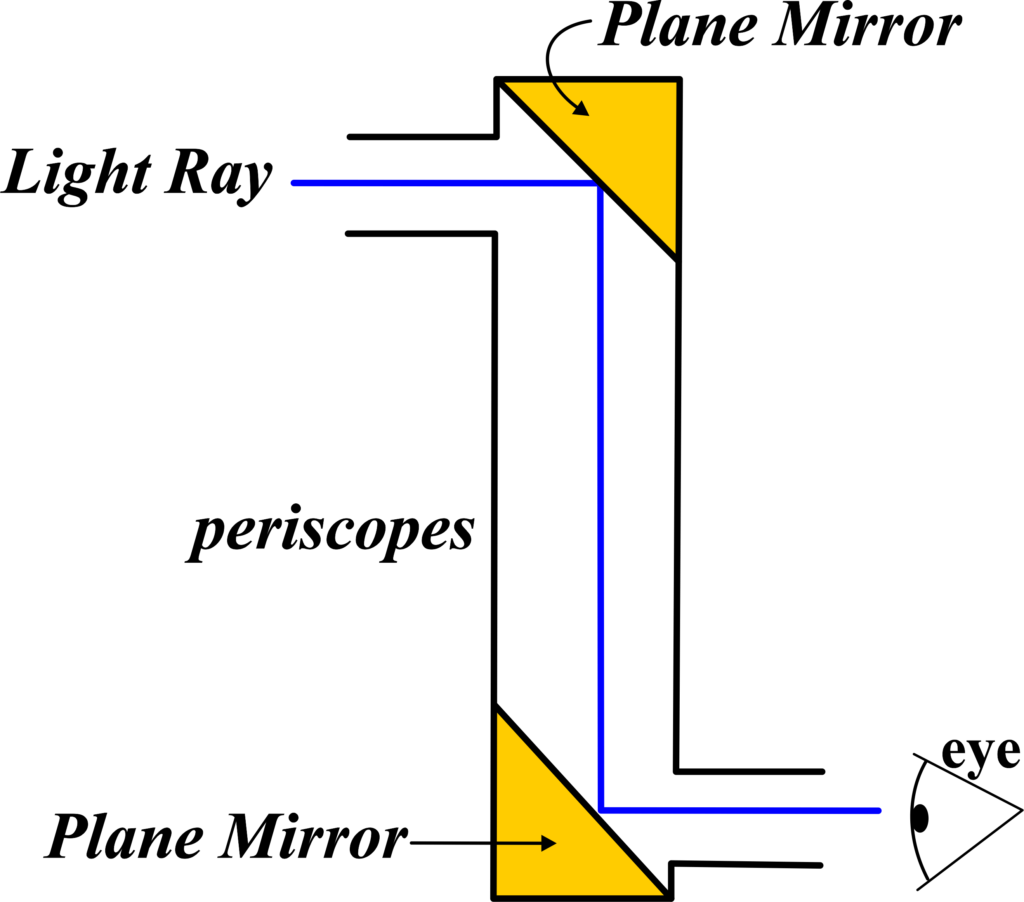
- Mirrors at 45° Angles: A periscope consists of an outer case with two mirrors placed at each end. These mirrors are set parallel to each other and at a 45° angle.
- Path of Light: When light from an object strikes the top mirror, it is reflected down the length of the periscope to the bottom mirror. Then, the bottom mirror reflects the light into the viewer’s eyes.
- No Magnification: A standard periscope does not magnify the image; it merely redirects the view. This allows the observer to see as if they were positioned at the top of the periscope.
- Uses: Periscopes are commonly used in submarines to allow the crew to see above the water’s surface while remaining submerged. They can also be used in other situations where direct viewing is obstructed.
A periscope uses the laws of reflection to extend the viewer’s line of sight, which is a practical application of basic optical principles. It’s a clear example of how angles and reflective surfaces can be used to manipulate the path of light for visual purposes.
Applications of Optical Instruments
Optical instruments have a wide range of applications, including in medicine, astronomy, research, and everyday life for vision correction.
Scientific Research: In scientific research, optical instruments like microscopes and telescopes are indispensable. Microscopes allow scientists to observe the smallest structures, such as cells and bacteria, leading to breakthroughs in biology and medicine. Telescopes enable astronomers to explore distant galaxies, stars, and planets, expanding our understanding of the universe.
Medical Field: In the medical field, optical instruments are used for diagnostics and surgeries. Endoscopes, for example, allow doctors to look inside the human body without invasive surgery. Lasers, which are also optical devices, are used for precise surgical procedures and treatments.
Communication Technology: Optical fibers, which are thin strands of glass or plastic, transmit data at the speed of light and are the backbone of modern communication systems. They are used for internet connections, telephone lines, and cable television.
Everyday Use: For everyday use, optical instruments like glasses and contact lenses correct vision defects, while cameras capture and preserve our memories. Binoculars and magnifying glasses help us see things more clearly and in greater detail.
Entertainment and Art: In entertainment, projectors and laser shows rely on optics to create visual spectacles. Kaleidoscopes, with their beautiful patterns of light, are both toys and artistic devices.
Navigation and Safety: For navigation and safety, periscopes are used in submarines to observe the surface of the water from below, and lighthouses use powerful lenses to guide ships safely to shore.
These applications demonstrate the versatility of optical instruments and their profound impact on our ability to explore, understand, and interact with the world around us. For students, recognizing these applications highlights the practical importance of optics in their studies and future careers.
Solved Examples
Example 1: Calculate the power of the lens required for a person with a near point of 50 cm to read a book comfortably at 25 cm.
Solution: The power of the lens (P) is given by:
\(\displaystyle P = \frac{1}{f}\)
The person wants to see clearly at 25 cm instead of 50 cm. So, we need to use the lens formula for near vision correction:
\(\displaystyle\frac{1}{f} = \frac{1}{d} – \frac{1}{D}\)
(d) = desired near point (25 cm or 0.25 m); (D) = actual near point (50 cm or 0.5 m)
Substitute the values:
$latex \displaystyle\frac{1}{f} = \frac{1}{0.25} – \frac{1}{0.5}
$latex \displaystyle\frac{1}{f} = 4 – 2 = 2 \, \text{m}^{-1}
The power of the lens required is +2 diopters.
Example 2: A simple microscope has a focal length of 5 cm. Calculate the magnifying power when the final image is formed at the near point of 25 cm.
Solution: The magnifying power (M) of a simple microscope is given by:
\(\displaystyle M = 1 + \frac{D}{f}\)
(D) = least distance of distinct vision (25 cm); (f) = focal length of the lens (5 cm)
Substitute the values:
$latex \displaystyle M = 1 + \frac{25}{5}
$latex \displaystyle M = 1 + 5 = 6
The magnifying power of the simple microscope is 6.
Example 3: A compound microscope has an objective lens of focal length 1 cm and an eyepiece of focal length 5 cm. The object is placed 1.2 cm from the objective lens. Calculate the total magnification if the final image is at infinity.
Solution: For a compound microscope, the total magnification (M) is the product of the magnifications of the objective lens (Mo) and the eyepiece (Me).
The magnification of the objective lens (Mo) is:
\(\displaystyle M_o = \frac{v_o}{u_o}\)
Using the lens formula for the objective lens:
\(\displaystyle\frac{1}{f_o} = \frac{1}{v_o} – \frac{1}{u_o}\)
(fo = 1 cm) (uo = -1.2 cm)
Substitute the values:
\(\displaystyle\frac{1}{1} = \frac{1}{v_o} – \frac{1}{-1.2}\)
\(\displaystyle 1 = \frac{1}{v_o} + \frac{1}{1.2}\)
\(\displaystyle 1 = \frac{1 + 1.2}{v_o \cdot 1.2}\)
\(\displaystyle 1 = \frac{2.2}{1.2 v_o}\)
\(\displaystyle v_o = \frac{2.2}{1.2} = \frac{11}{6} \approx 1.83 \, \text{cm}\)
Therefore, the magnification of the objective lens (Mo) is:
\(\displaystyle M_o = \frac{v_o}{u_o} = \frac{1.83}{1.2} \approx 1.525\)
The magnification of the eyepiece (Me) for a relaxed eye (final image at infinity) is:
\(\displaystyle M_e = \frac{D}{f_e}\)
(D = 25 cm); (fe = 5 cm)
\(\displaystyle M_e = \frac{25}{5} = 5\)
Therefore, the total magnification \(M\) is:
\(\displaystyle M = M_o \cdot M_e = 1.525 \times 5 = 7.625\)
The total magnification of the compound microscope is approximately 7.625.
Example 4: An astronomical telescope has an objective lens of focal length 100 cm and an eyepiece of focal length 5 cm. Calculate the magnifying power of the telescope when the final image is formed at infinity.
Solution: The magnifying power (M) of an astronomical telescope when the final image is at infinity is given by:
\(\displaystyle M = -\frac{f_o}{f_e}\)
(fo = 100 cm); (fe = 5 cm)
Substitute the values:
\(\displaystyle M = -\frac{100}{5} = -20\)
The negative sign indicates that the image is inverted. The magnifying power of the astronomical telescope is 20 (inverted).
FAQs
What are optical instruments and why are they important?
Optical instruments are devices that use lenses, mirrors, or a combination of both to manipulate light to enhance the visual perception of objects. They are important because they allow us to see details that are otherwise too small, too distant, or otherwise difficult to observe with the naked eye. Examples include microscopes, telescopes, cameras, and eyeglasses.
How does the human eye function as an optical instrument?
The human eye functions as an optical instrument by focusing light onto the retina using a convex lens. Light enters the eye through the cornea, and passes through the aqueous humor, the pupil, and the lens, which adjusts its shape to focus the light. The focused light forms an image on the retina, which is then converted into electrical signals and interpreted by the brain.
What is a microscope and how does it work?
A microscope is an optical instrument that magnifies small objects, allowing us to see details not visible to the naked eye. It typically consists of an objective lens and an eyepiece lens. The objective lens forms an enlarged image of the object, and the eyepiece lens further magnifies this image. This combination allows for high levels of magnification.
How is magnification in a microscope calculated and what factors affect it?
Magnification in a microscope is calculated by multiplying the magnifying power of the objective lens by that of the eyepiece lens. Factors affecting magnification include the focal lengths of these lenses and the distance between them. Additionally, the quality and alignment of the lenses can affect the clarity and detail of the magnified image.
What is a telescope and how does it differ from a microscope?
A telescope is an optical instrument designed to observe distant objects, such as stars and planets. It typically uses lenses or mirrors to collect and focus light from these distant objects. Unlike a microscope, which magnifies small, close objects, a telescope magnifies large, far-away objects by collecting more light and reducing the angle of vision, making distant objects appear closer and clearer.
How does a kaleidoscope create its patterns, and what principles of optics are involved?
A kaleidoscope creates patterns through the reflection of light inside a tube containing mirrors and colored objects. When viewed through one end, the multiple reflections of the objects create symmetrical, colorful patterns. The principles of reflection and symmetry in optics are key to how a kaleidoscope works, as light bounces off the mirrors to create intricate designs.
What is a periscope and how is it used?
A periscope is an optical instrument that allows an observer to see over or around obstacles. It consists of a tube with mirrors or prisms at each end set at 45-degree angles. Light from the object reflects off the top mirror down the tube to the bottom mirror, which then reflects it to the observer’s eye. Periscopes are commonly used in submarines and by soldiers in trenches to see their surroundings without being exposed.