Wave Nature of Matter

The story begins in the early 20th century. Scientists were exploring the nature of light and matter. They discovered that light, which was always thought to be a wave, also showed particle-like properties (as seen in the photoelectric effect). This led to the question: If light can be both a wave and a particle, could matter, typically thought of as particles, also have wave-like properties?

Physics at the turn of the 20th century was in a state of flux. The discovery of the electron, the understanding of atomic structure, and the behavior of light led to more questions than answers. Scientists were beginning to realize that the laws of classical physics couldn’t explain the strange world of the very small.

The first hint that matter might have wave-like properties came from the study of light. Experiments showed that light behaved like a wave, spreading out and creating interference patterns. But then, the photoelectric effect demonstrated that light also behaved like a particle, knocking electrons off metal surfaces. This dual nature was puzzling.

In 1924, a young French physicist named Louis de Broglie made a groundbreaking proposal. He suggested that if light, which was known to be a wave, could also behave like a particle, then perhaps particles of matter could also behave like waves. This was a radical idea because it suggested a fundamental symmetry in nature.

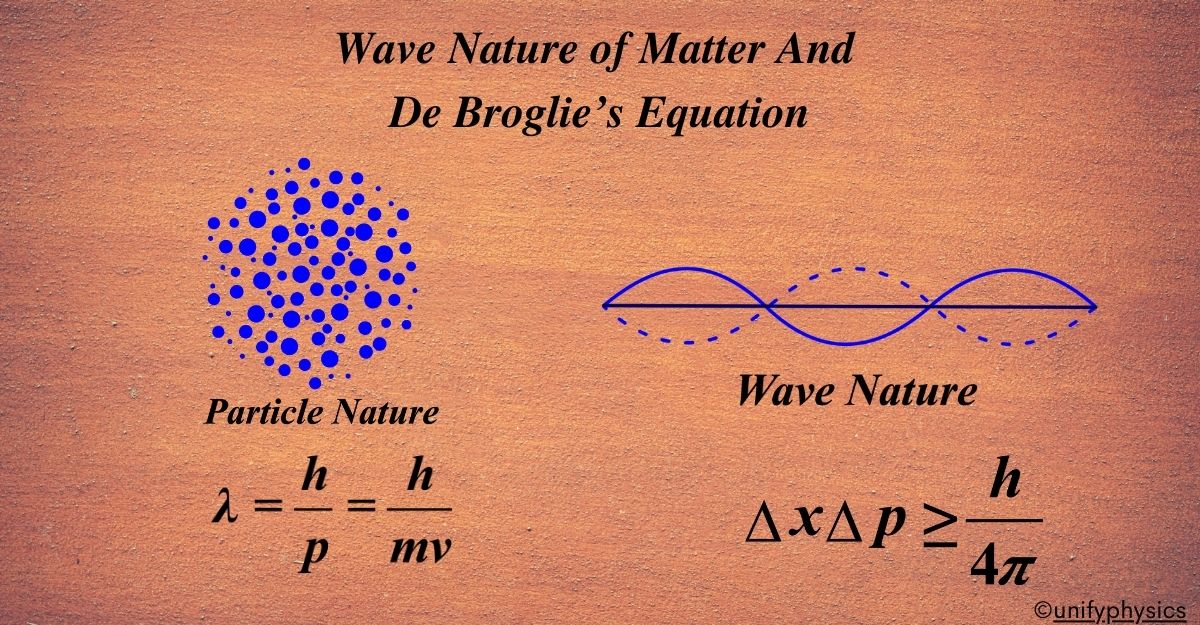
De Broglie proposed that the wavelength of a particle was related to its momentum (the product of its mass and velocity). He expressed this relationship through the equation: \(\displaystyle\lambda = \frac{h}{mv}\) This simple equation linked the macroscopic world we can see with the microscopic quantum world.

The true test of De Broglie’s hypothesis came with experiments. The Davisson-Germer experiment in 1927 confirmed that electrons could produce interference patterns, just like waves. This was direct evidence of the wave nature of matter.

De Broglie’s work laid the foundation for quantum mechanics, a new branch of physics that deals with the behavior of particles at the atomic and subatomic levels. Quantum mechanics has since become one of the most successful theories in physics, explaining the behavior of atoms and the particles that make them up.

Building on the wave-particle duality, Werner Heisenberg introduced the Uncertainty Principle in 1927. This principle states that it is impossible to measure both the position and momentum of a particle with absolute precision. The more precisely one is known, the less precise the measurement of the other is. This principle is a fundamental limit on our ability to predict the behavior of quantum systems.

The wave nature of matter and the principles of quantum mechanics have profound implications. They are essential for the technology that powers our computers, smartphones, and medical imaging devices. They also challenge our classical intuitions about how the world works at a fundamental level.

Wave Nature of Matter

Consider an experiment with electrons, which are tiny particles that orbit the nucleus of an atom. When electrons are fired through a double-slit apparatus, they create an interference pattern on a screen placed behind the slits. This pattern of alternating bright and dark bands is characteristic of waves, not particles. If electrons were simply particles, we would expect them to form two distinct clusters behind the slits, but instead, they create a pattern similar to that of light waves passing through the same setup.

This experiment shows that electrons, which are matter, exhibit wave-like behavior. The interference pattern is a result of the waves associated with each electron combining—where the waves reinforce each other, we see bright bands, and where they cancel each other out, we see dark bands. This wave behavior is not limited to electrons; it applies to all matter, although it is most noticeable in particles with very small mass, like electrons.

This wave behavior is not limited to electrons; it applies to all matter, although it is most noticeable in particles with very small mass, like electrons. The wave nature of matter is a fundamental concept in quantum mechanics and has been confirmed by numerous experiments. It tells us that the universe at its smallest scale doesn’t behave at all like the world we see around us.

Imagine you’re at the beach, watching waves crash onto the shore. These waves carry energy and move through the water. Now, what if I told you that particles of matter, like electrons, can behave like those waves you see at the beach? That’s the essence of the wave nature of matter.

In the early 20th century, scientists discovered that particles of matter could exhibit wave-like properties. This was a surprising discovery because we usually think of matter as having mass and taking up space, not spreading out and creating patterns like waves do.

So, how do we know matter can behave like waves? Well, when particles like electrons pass through a narrow opening or across an edge, they create patterns that are very similar to those created by waves when they encounter obstacles. These patterns are called interference patterns and are a hallmark of wave behavior.

This wave-like behavior of matter has some interesting consequences. For example, it means that there are certain things about particles that we can’t know for sure, like exactly where they are at any given moment. Instead, we can only talk about the probability of finding a particle in a particular place.

When we talk about the wave nature of matter, we’re saying that particles aren’t just little balls moving around; they also have properties like waves. This dual nature is one of the fundamental ideas in physics and has led to many advances in technology and our understanding of the universe.

De Broglie’s Hypothesis

De Broglie’s hypothesis stated that all moving particles—electrons, atoms, even baseballs—have a wave associated with them. However, for everyday objects like baseballs, this wavelength is incredibly tiny and thus, not observable. Imagine you’re at a concert, and you can feel the music vibrating through you. These vibrations are waves of sound. Now, what if I told you that particles, like the electrons in the atoms of your body, also have their own kind of “music” or waves? This is the core idea behind De Broglie’s Hypothesis.

In 1924, a French physicist named Louis de Broglie proposed a revolutionary idea: just like light, which was known to have wave-like properties, matter also has wave-like properties. He suggested that particles of matter, such as electrons, could be described not just as particles moving through space but also as waves that spread out and interfere with each other.

De Broglie’s Hypothesis was a bold leap because it combined the concepts of particles, which have mass, with the concept of waves, which traditionally didn’t. It bridged the gap between the physics of the large (classical mechanics) and the physics of the very small (quantum mechanics).

The hypothesis states that any particle of matter that has momentum (which is the product of mass and velocity) also has a wavelength associated with it. This wavelength is called the “de Broglie wavelength.” The idea is that the faster a particle is moving, the shorter its wavelength will be, and vice versa.

This concept was groundbreaking because it introduced a new way of looking at the behavior of particles at the atomic and subatomic levels. It led to the understanding that at these tiny scales, matter doesn’t behave at all like what we see in our everyday lives. Instead, it has this dual nature, sometimes acting like a particle, and sometimes like a wave.

Imagine you’re playing with a toy car. The faster you push it, the more momentum it has, and the harder it is to stop. Now, what if I told you that particles like electrons also have something similar to momentum, and this momentum is linked to a wave-like property? That’s what De Broglie’s Equation is all about.

De Broglie’s Equation connects the momentum of a particle with its wave-like nature. The equation is simple yet profound:

\(\displaystyle\lambda = \frac{h}{p}\)

• (λ) is the wavelength of the particle’s wave.
• (h) is Planck’s constant, a very important number in quantum physics.
• (p) is the momentum of the particle, which is the product of its mass (m) and velocity (v).

If you know the momentum of a particle, you can use De Broglie’s Equation to find out the wavelength of the wave associated with it. This is cool because it tells us that particles at the quantum level, like electrons zipping around an atom, don’t just move like little bullets; they also have wave-like properties that can be described mathematically.

Planck’s constant, denoted by (h), is a fundamental physical constant that is of great importance in quantum mechanics. It relates the energy of a photon to its frequency and also connects the wavelength of a matter wave to the momentum of the associated particle. The value of Planck’s constant is defined as exactly ( 6.62607015 × 10-³⁴) joule seconds (J⋅s).

In simple terms, Planck’s constant is the bridge between the macroscopic world we can see and the microscopic quantum world. It’s a key part of the equations that describe how light and matter behave at the smallest scales. For example, the energy (E) of a photon is calculated by multiplying the frequency (ν) of the electromagnetic wave by Planck’s constant: ( E = hν).

Max Planck introduced this constant in 1900 while working on black-body radiation, and it has since become a cornerstone of quantum physics, influencing our understanding of phenomena like the photoelectric effect and the wave nature of particles.

De Broglie’s equation is deeply connected to the double-slit experiment, which is a classic demonstration of the wave-particle duality of matter. Here’s how they relate:

It predicts that every particle has a wave associated with it, and the wavelength (λ) of this wave is given by the equation:

\(\displaystyle\lambda = \frac{h}{p}\)

where (h) is Planck’s constant and (p) is the momentum of the particle.

In this experiment, particles such as electrons are fired at a barrier with two slits. If particles were only particles, they would pass through the slits and form two distinct clusters on a screen behind the barrier. However, what’s observed is an interference pattern of alternating bright and dark bands, which is characteristic of waves, not particles.

The interference pattern observed in the double-slit experiment is a direct result of the wave nature of particles predicted by De Broglie. The waves associated with each particle passing through the slits interfere with each other, creating the pattern. The wavelength of these matter waves, as given by De Broglie’s equation, determines the spacing and structure of the interference pattern.

The double-slit experiment with electrons confirms De Broglie’s hypothesis. When the electrons pass through the slits, they behave like waves, and their wave-like nature is described by the wavelength calculated using De Broglie’s equation. This experimentally demonstrates that matter does exhibit wave properties, just as De Broglie proposed.

Heisenberg’s Uncertainty Principle

Werner Heisenberg, another giant in physics, introduced a principle that complements De Broglie’s ideas. He stated that it’s impossible to know a particle’s position and momentum precisely at the same time. The more accurately you know one, the less accurately you can know the other.

Imagine you’re experimenting to observe an electron, a fundamental particle in atoms. Electrons are tiny and exhibit both particle-like and wave-like behavior. To observe the electron’s position, you might use a photon of light, which is also a particle with wave-like properties.

According to Heisenberg’s Uncertainty Principle, the more precisely you try to measure the position of the electron, the more uncertain you become of its momentum. This is because the photon used to observe the electron will inevitably interact with it, altering its momentum. It’s like trying to measure the position of a tiny ball by hitting it with another ball; the collision will change the speed and direction of the tiny ball, making it impossible to know its momentum accurately at the same time.

This principle can be expressed mathematically as:

\(\displaystyle\Delta x \cdot \Delta p \geq \frac{h}{4\pi}\)

where (∆x) is the uncertainty in position, (∆p) is the uncertainty in momentum, and (h) is Planck’s constant.

Think of a birthday balloon filled with helium, floating in a room. You can gently tap the balloon to send it drifting in one direction or another. Now, let’s say you want to measure two things: the exact position of the balloon in the room and the speed at which it’s moving after you tap it.

If you focus on finding the exact position of the balloon, you can do so by taking a snapshot with a camera. However, the balloon appears frozen in that still photo, so you can’t tell how fast it’s moving or in what direction.

On the other hand, if you want to measure how fast the balloon is moving, you could use a video camera to capture its motion. But because the balloon is constantly moving, the video won’t show you a single, precise location of the balloon at any given moment.

Heisenberg’s Uncertainty Principle is like trying to take both the snapshot and the video at the same time. The more you try to pin down where the balloon is (the snapshot), the less you know about its speed and direction (the video), and vice versa. This principle tells us that at the quantum level, nature has a built-in fuzziness, and we have to accept that some things just can’t be known with complete precision at the same time.

Also Read: Photoelectric Effect

Solved Examples

Problem 1: Calculate the de Broglie wavelength of an electron moving with a velocity of (\(\displaystyle 2 \times 10^6 m/s\)). (Given: mass of electron ( \(\displaystyle m = 9.11 \times 10^{-31} kg\)), Planck’s constant ( \(\displaystyle h = 6.63 \times 10^{-34} Js\)).

Solution: The de Broglie wavelength (λ) is given by:

\(\displaystyle\lambda = \frac{h}{mv}\)

Substitute the given values:

\(\displaystyle\lambda = \frac{6.63 \times 10^{-34}}{9.11 \times 10^{-31} \times 2 \times 10^6}\)

\(\displaystyle\lambda = \frac{6.63 \times 10^{-34}}{1.822 \times 10^{-24}}\)

\(\displaystyle\lambda = 3.64 \times 10^{-10} \, \text{m}\)

The de Broglie wavelength of the electron is (3.64 ×10^-10 m).

Problem 2: An electron is confined within a region of width (0.1 nm). Calculate the minimum uncertainty in its momentum. (Given: Planck’s constant ( \(\displaystyle h = 6.63 \times 10^{-34} \, \text{Js} \)).

Solution: Using Heisenberg’s Uncertainty Principle:

\(\displaystyle\Delta x \cdot \Delta p \geq \frac{h}{4\pi}\)

Given ( \(\displaystyle\Delta x = 0.1 \, \text{nm} = 0.1 \times 10^{-9} \, \text{m} \)):

\(\displaystyle\Delta p \geq \frac{6.63 \times 10^{-34}}{4\pi \times 0.1 \times 10^{-9}}\)

\(\displaystyle\Delta p \geq \frac{6.63 \times 10^{-34}}{1.256 \times 10^{-9}}\)

\(\displaystyle\Delta p \geq 5.28 \times 10^{-25} \, \text{kg m/s}\)

The minimum uncertainty in the momentum of the electron is (\(\displaystyle 5.28 \times 10^{-25} \, \text{kg m/s}\)).

Problem 3: A photon has an energy of (3 eV). Calculate its de Broglie wavelength. (Given: (1 eV= \(\displaystyle 1.6 \times 10^{-19} \, \text{J}\)), Planck’s constant ( \(\displaystyle h = 6.63 \times 10^{-34} \, \text{Js} \)), speed of light ( \(\displaystyle c = 3 \times 10^8 \, \text{m/s} \)).

Solution: First, convert the energy from eV to joules:

\(\displaystyle E = 3 \times 1.6 \times 10^{-19} = 4.8 \times 10^{-19} \, \text{J}\)

For a photon, the energy (E) is related to its wavelength (λ) by:

\(\displaystyle E = \frac{hc}{\lambda}\)

Rearrange to solve for (λ):

\(\displaystyle\lambda = \frac{hc}{E}\)

Substitute the given values:

\(\displaystyle\lambda = \frac{6.63 \times 10^{-34} \times 3 \times 10^8}{4.8 \times 10^{-19}}\)

\(\displaystyle\lambda = \frac{1.989 \times 10^{-25}}{4.8 \times 10^{-19}}\)

\(\displaystyle\lambda = 4.14 \times 10^{-7} \, \text{m} = 414 \, \text{nm}\)

The de Broglie wavelength of the photon is (414 nm).

Problem 4: A proton is moving with a speed of (\(\displaystyle 5 \times 10^6 \, \text{m/s}\)) with an uncertainty of (0.01 %). Calculate the uncertainty in its position. (Given: mass of proton ( \(\displaystyle m_p = 1.67 \times 10^{-27} \, \text{kg} \)), Planck’s constant ( \(\displaystyle h = 6.63 \times 10^{-34} \, \text{Js} \)).

Solution: First, calculate the uncertainty in momentum (∆p):

\(\displaystyle\Delta v = 0.01\% \times 5 \times 10^6 = 0.0001 \times 5 \times 10^6 = 500 \, \text{m/s}\)

\(\displaystyle\Delta p = m_p \Delta v = 1.67 \times 10^{-27} \times 500 = 8.35 \times 10^{-25} \, \text{kg m/s}\)

Using Heisenberg’s Uncertainty Principle:

\(\displaystyle\Delta x \cdot \Delta p \geq \frac{h}{4\pi}\)

\(\displaystyle\Delta x \geq \frac{6.63 \times 10^{-34}}{4\pi \times 8.35 \times 10^{-25}}\)

\(\displaystyle\Delta x \geq \frac{6.63 \times 10^{-34}}{2.63 \times 10^{-24}}\)

\(\displaystyle\Delta x \geq 2.52 \times 10^{-10} \, \text{m}\)

The uncertainty in the position of the proton is (\(\displaystyle 2.52 \times 10^{-10} \, \text{m}\)).

Problem 5: Calculate the de Broglie wavelength of a baseball of mass (0.145 kg) moving with a velocity of (40m/s). (Given: Planck’s constant (\(\displaystyle h = 6.63 \times 10^{-34} \, \text{Js} \)).

Solution: The de Broglie wavelength (λ) is given by:

\(\displaystyle\lambda = \frac{h}{mv}\)

Substitute the given values:

\(\displaystyle\lambda = \frac{6.63 \times 10^{-34}}{0.145 \times 40}\)

\(\displaystyle\lambda = \frac{6.63 \times 10^{-34}}{5.8} = 1.14 \times 10^{-34} \, \text{m}\)

The de Broglie wavelength of the baseball is (\(\displaystyle 1.14 \times 10^{-34} \, \text{m}\)).

Problem 6:  An unstable particle has an average lifetime of (\(\displaystyle 1 \times 10^{-10} \, \text{s}\)). Calculate the minimum uncertainty in its energy. (Given: Planck’s constant ( \(\displaystyle h = 6.63 \times 10^{-34} \, \text{Js} \)).

Solution: Using the energy-time form of Heisenberg’s Uncertainty Principle:

\(\displaystyle\Delta E \cdot \Delta t \geq \frac{h}{4\pi}\)

Given ( \(\displaystyle\Delta t = 1 \times 10^{-10} \, \text{s} \)):

\(\displaystyle\Delta E \geq \frac{6.63 \times 10^{-34}}{4\pi \times 1 \times 10^{-10}}\)

\(\displaystyle\Delta E \geq \frac{6.63 \times 10^{-34}}{1.256 \times 10^{-10}} = 5.28 \times 10^{-24} \, \text{J}\)

Convert the energy to electron volts:

\(\displaystyle\Delta E = \frac{5.28 \times 10^{-24}}{1.6 \times 10^{-19}} \approx 3.3 \times 10^{-5} \, \text{eV}\)

The minimum uncertainty in the energy of the particle is (\(\displaystyle 3.3 \times 10^{-5} \, \text{eV}\)).

FAQs