The story of the Zeroth Law of Thermodynamics is unique. It’s a fundamental principle that was recognized after the first three laws of thermodynamics were already known. The name “Zeroth” comes from the fact that it needed to be placed before the First Law due to its foundational nature, even though it was discovered later.
Ralph H. Fowler, a British physicist, introduced the term “Zeroth Law” in the 1930s. Before this law was formulated, scientists had already established the First, Second, and Third Laws of Thermodynamics, which dealt with energy, entropy, and absolute zero, respectively. However, they realized something was missing: a law that could define temperature clearly and scientifically.
The Zeroth Law filled this gap by providing a precise definition of temperature. It essentially says that if two systems are in thermal equilibrium with a third system, then they must be in thermal equilibrium with each other. This might seem obvious now, but at the time, it was a groundbreaking concept that allowed scientists to measure temperature consistently and compare it between different systems.
What Is the Zeroth Law of Thermodynamics?
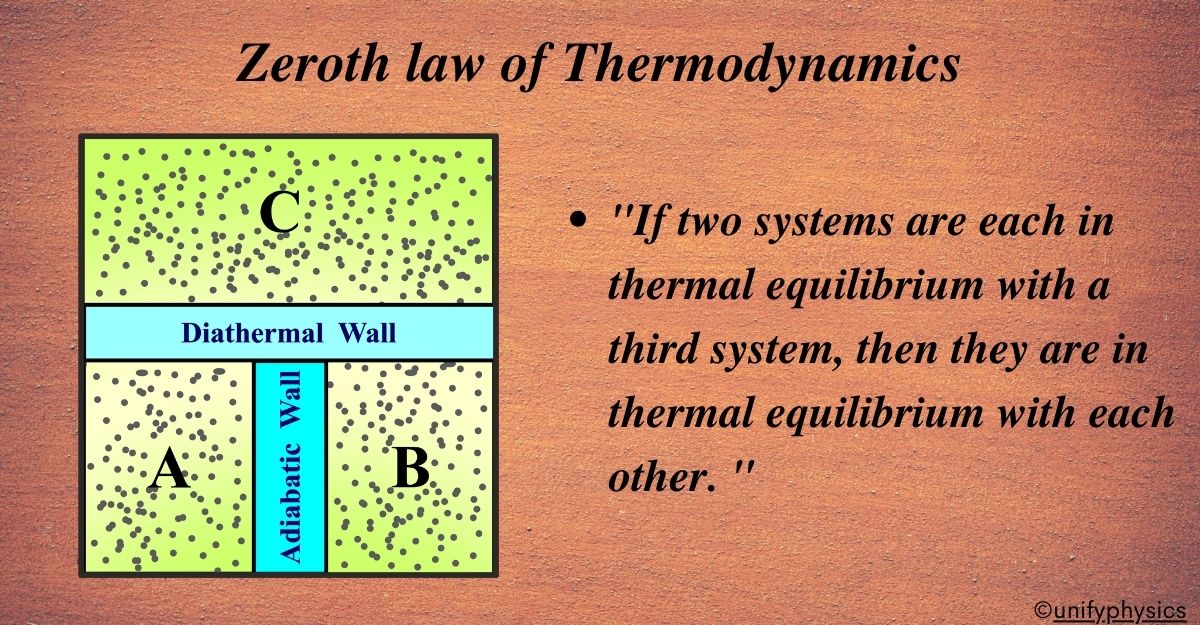
The Zeroth Law of Thermodynamics states: “If two systems are each in thermal equilibrium with a third system, then they are in thermal equilibrium with each other. “
Imagine you have three cups of water: one hot, one warm, and one cold. If you put the hot cup next to the warm cup, the hot water will make the warm water hotter, right? And if you put the warm cup next to the cold one, the warm water will make the cold water warmer. But what if you put the hot cup next to the cold one? What happens then?

Well, the Zeroth Law says that if you wait long enough, the hot water will cool down, and the cold water will warm up until they both reach a point where they don’t change temperature anymore. This point is called thermal equilibrium. In simpler terms, it means they both become the same temperature!
So, the Zeroth Law tells us that if two things are in thermal equilibrium with a third thing, then they’re also in thermal equilibrium with each other. It’s like saying if two people have the same friend, they’re likely to be friends with each other too.
Also Read: Calorimetry
Thermal Equilibrium
Thermal equilibrium occurs when two systems have been in contact long enough to equalize their temperatures, and no heat flows between them. It’s a state where all parts of the systems have the same temperature.
Imagine you’re organizing a get-together where everyone is asked to bring a dish that’s served hot. Now, you have a big table where all these dishes are placed. As time passes, the hot dishes start cooling down, and the room-temperature dishes start warming up a bit. After a while, all the dishes reach a point where they feel the same in terms of temperature. This is what we call thermal equilibrium.
In physics, thermal equilibrium is a condition where two or more objects that are in contact with each other reach a common temperature after a certain period. This means that there is no net heat flow between them anymore. Heat always flows from the hotter object to the cooler one until they both reach the same temperature.
For example, if you take a hot mug of coffee and leave it on your desk, it will eventually cool down to match the room’s temperature. The mug and the room are in thermal equilibrium because there is no longer any heat transfer between them.
This concept is crucial because it forms the basis of how we understand temperature and heat flow. It’s also why we can use thermometers to measure temperature. When we put a thermometer in contact with an object, we wait for it to reach thermal equilibrium with the object. The temperature reading we get is the result of this equilibrium.
Zeroth Law of Thermodynamics Example
Imagine three separate systems—System A, System B, and System C—each with its energy content. System A and System C are separated by a diathermal wall that allows heat to pass through, while System B and System C are also connected by a similar diathermal wall. However, there’s no direct diathermal wall between System A and System B, meaning they can’t exchange heat directly.
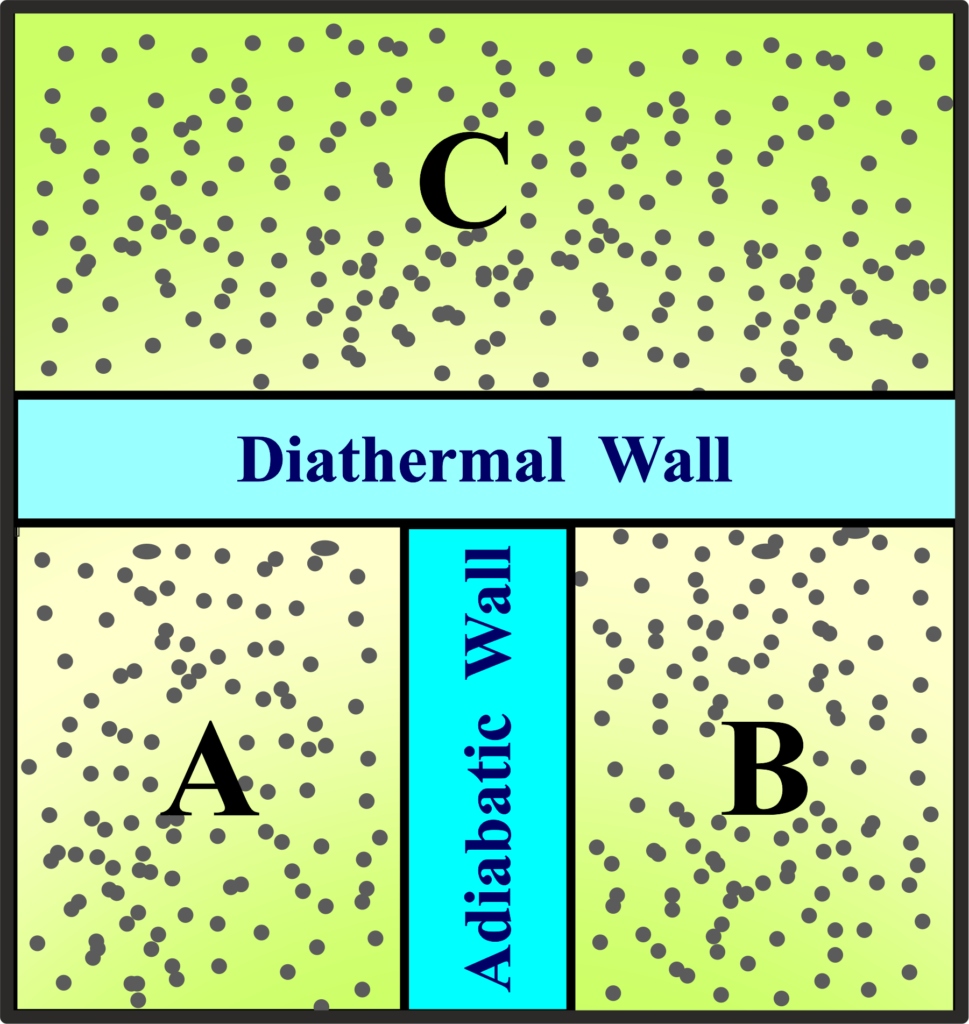
Now, let’s say System A and System C have been in contact for a while, and they’ve reached a point where no heat flows between them anymore; they are in thermal equilibrium. The same goes for System B and System C.
According to the Zeroth Law of Thermodynamics, if System A is in thermal equilibrium with System C, and System B is also in thermal equilibrium with System C,
then System A and System B must be in thermal equilibrium with each other. This means that even though System A and System B aren’t directly connected, they still end up having the same temperature because they both have the same temperature as System C.
This law is fundamental because it allows us to define temperature in a precise way. It’s the reason we can use thermometers to measure temperature reliably and why we can say with confidence that two systems in equilibrium with a third system are also in equilibrium with each other. It’s like a chain of temperature connections—once two systems are connected through a third system, they’re effectively connected as well.
Now we look into another example; Imagine you’re at a science fair, and there’s a demonstration with three cups of water, each labeled A, B, and C. The cups are used to explain the Zeroth Law of Thermodynamics in a way everyone can understand, especially 12th-grade students.
Example: Cup A is filled with water at a comfortable 30°C, similar to a pleasant day outside. Cup B has water at a warmer 40°C, like a hot summer afternoon. Cup C also has water at 30°C, just like Cup A.
- Cup A and Cup C: These two are already friends in terms of temperature. They’re both at 30°C, so they’re in thermal equilibrium. No heat flows between them because they’re equally warm.
- Cup B Meets Cup C: Cup B, which is warmer, is brought into contact with Cup C. Since Cup C is cooler, heat from Cup B starts to flow into Cup C. They’re having a temperature conversation, trying to reach an agreement.
- Reaching Equilibrium: After some time, Cup B cools down, and Cup C warms up a bit until they both settle at 30°C. They’ve reached an understanding and are now in thermal equilibrium.
- The Zeroth Law in Action: Now, according to the Zeroth Law, since Cup A was in thermal equilibrium with Cup C, and Cup B reached thermal equilibrium with Cup C, Cup A, and Cup B must be in thermal equilibrium with each other. They didn’t need to be directly compared or mixed; the law tells us they’re the same temperature because they both agreed with Cup C.
This example helps students visualize how the Zeroth Law works. It’s like a social network where if two people are friends with the same person, they’re likely to be friends too. In the world of thermodynamics, this friendship is all about temperature balance.
Application of Zeroth Law of Thermodynamics
This law is crucial for the practical use of thermometers. When we measure temperature, we rely on the Zeroth Law. For instance, when a thermometer is in thermal equilibrium with a system, it shows its temperature, which will be the same as any other system in equilibrium.
Thermometers: A thermometer is a device that measures temperature. It works because of the Zeroth Law.
- When you’re feeling unwell, and you put a thermometer under your tongue, the thermometer’s liquid (usually mercury or colored alcohol) starts at room temperature.
- As it sits there, heat from your body transfers to the thermometer until they’re both at the same temperature—this is thermal equilibrium.
- The liquid inside the thermometer expands and rises up the tube as it warms up. The scale next to the tube tells you what your body temperature is.
This is the Zeroth Law in action. The thermometer reaches thermal equilibrium with your body, and because of the Zeroth Law, we know that the temperature reading on the thermometer is the same as your body’s temperature.
Predicting Heat Flow: The Zeroth Law also helps us predict how heat will move between objects. For example, if you put a hot mug on a cold coaster, the Zeroth Law tells us that eventually, the mug and the coaster will end up at the same temperature because they’ll reach thermal equilibrium.
Engineering and Design: In engineering, the Zeroth Law is used to design systems that control temperature, like air conditioners and refrigerators. Engineers need to understand how different materials and components will come to the same temperature to make these systems efficient.
Scientific Research: In scientific research, the Zeroth Law allows scientists to compare temperatures in experiments accurately. Whether they’re studying the effects of temperature on chemical reactions or the behavior of materials at different temperatures, the Zeroth Law ensures that their temperature measurements are consistent and reliable.
Solved Examples
Problem 1: Three systems A, B, and C are such that A is in thermal equilibrium with B and B is in thermal equilibrium with C. If the temperature of A is 300 K, what is the temperature of C?
Solution: According to the Zeroth Law of Thermodynamics, if two systems are each in thermal equilibrium with a third system, they are in thermal equilibrium with each other. Therefore, the temperature of C must be the same as that of A.
The temperature of C is 300 K.
Problem 2: A thermometer is calibrated such that it reads 0°C when in thermal equilibrium with melting ice and 100°C when in thermal equilibrium with boiling water at standard atmospheric pressure. If this thermometer is in thermal equilibrium with another system and reads 50°C, what can be concluded about the system’s temperature?
Solution: The thermometer is properly calibrated and shows the temperature directly. If it reads 50°C, the system it is in equilibrium with must also be at 50°C.
The system’s temperature is 50°C.
Problem 3: Two bodies, one at 400 K and the other at 300 K, are placed in thermal contact. Assuming no heat loss to the surroundings and equal heat capacities for both bodies, find the final equilibrium temperature.
Solution: When two bodies with equal heat capacities are brought into thermal contact, the final temperature will be the average of their initial temperatures.
\(\displaystyle T_{\text{final}} = \frac{T_1 + T_2}{2} = \frac{400 \, \text{K} + 300 \, \text{K}}{2} = 350 \, \text{K} \)
The final equilibrium temperature is 350 K.
Problem 4: A composite system is made up of two systems, A and B, with temperatures (TA) and (TB) respectively. If (TA = TB), what can be said about the energy exchange between the two systems?
Solution: According to the Zeroth Law of Thermodynamics, if (TA = TB), then systems A and B are in thermal equilibrium. There will be no net energy exchange between the two systems.
There is no net energy exchange between the two systems.
Problem 5: Two rods, one of copper and one of aluminum, are each 1 m long and are initially at temperatures of 300 K and 400 K, respectively. They are brought into thermal contact at one end. Assuming they have equal cross-sectional areas and negligible heat loss to the surroundings, what will be the temperature at the point of contact when thermal equilibrium is reached?
Solution: Since the rods are of equal length and cross-sectional area and assuming equal thermal conductivity, the point of contact will reach the average temperature of the two initial temperatures.
\(\displaystyle T_{\text{contact}} = \frac{T_{\text{Cu}} + T_{\text{Al}}}{2} = \frac{300 \, \text{K} + 400 \, \text{K}}{2} = 350 \, \text{K} \)
The temperature at the point of contact when thermal equilibrium is reached is 350 K.
Problem 6: A 1 kg block of ice at 0°C is mixed with 1 kg of water at 80°C in an insulated container. Assuming no heat loss to the surroundings and latent heat of fusion for ice as ( 334 kJ/kg), what is the final temperature of the mixture?
Solution: First, calculate the amount of heat required to melt the ice:
\(\displaystyle Q_{\text{melt}} = m \times L_f = 1 \, \text{kg} \times 334 \, \text{kJ/kg} = 334 \, \text{kJ} \)
Calculate the amount of heat the water can lose while cooling to 0°C:
\(\displaystyle Q_{\text{cool}} = m \times c \times \Delta T = 1 \, \text{kg} \times 4.18 \, \text{kJ/kg}^\circ\text{C} \times (80 – 0) \, ^\circ\text{C} = 334.4 \, \text{kJ} \)
Since the heat required to melt the ice (334 kJ) is almost equal to the heat lost by the water (334.4 kJ), the final temperature of the mixture will be 0°C with a small amount of water and melted ice. The final temperature of the mixture is 0°C.
FAQs
What is the Zeroth Law of Thermodynamics?
The Zeroth Law of Thermodynamics states that if two systems are each in thermal equilibrium with a third system, then they are in thermal equilibrium with each other. This law provides the basis for the concept of temperature.
Why is it called the “Zeroth Law”?
It is called the Zeroth Law because it was formulated after the First and Second Laws of Thermodynamics, but it is considered fundamental and logical before them. It establishes the concept of temperature, which is essential for the other laws.
How does the Zeroth Law of Thermodynamics help in measuring temperature?
The Zeroth Law of Thermodynamics allows for the creation of thermometers. Since the law states that systems in thermal equilibrium have the same temperature, a thermometer can be calibrated to measure temperature by reaching equilibrium with the system being measured.
Can you give an example of the Zeroth Law of Thermodynamics in action?
An example of the Zeroth Law is when you place a thermometer in a cup of hot water. The thermometer will eventually reach thermal equilibrium with the water, indicating the water’s temperature. If the same thermometer is placed in another cup of hot water and shows the same reading, the two cups of water are at the same temperature.
How does the Zeroth Law relate to thermal equilibrium?
The Zeroth Law defines thermal equilibrium. It states that if two systems are each in thermal equilibrium with a third system, then they are in thermal equilibrium with each other. This means there is no net heat flow between them, indicating they are at the same temperature.
What role does the Zeroth Law play in thermodynamics?
The Zeroth Law plays a crucial role in thermodynamics by establishing the concept of temperature as a measurable and comparable property. It lays the foundation for defining and comparing the thermal states of different systems.
How does the Zeroth Law ensure the transitive property of thermal equilibrium?
The Zeroth Law ensures the transitive property of thermal equilibrium by stating that if system A is in thermal equilibrium with system B, and system B is in thermal equilibrium with system C, then system A is also in thermal equilibrium with system C. This allows the temperature to be a consistent and transitive measure of thermal equilibrium.