Introduction
The Universal Law of Gravitation, a groundbreaking principle formulated by Sir Isaac Newton (25 December 1642 – 20 March 1726/27) in the 17th century, stands as one of the cornerstones of classical physics and a monumental leap forward in our comprehension of the forces governing the cosmos. Unveiled in Newton’s seminal work “Philosophiae Naturalis Principia Mathematica” in 1687, this law articulates the fundamental force that governs the gravitational attraction between any two masses in the universe.
In simple terms, the Universal Law of Gravitation tells us that every object with mass attracts every other object with mass. The strength of this attraction depends on the masses of the objects and the distance between them. This concept not only explains why a dropped object falls to the ground but also governs the majestic motions of planets in our solar system.
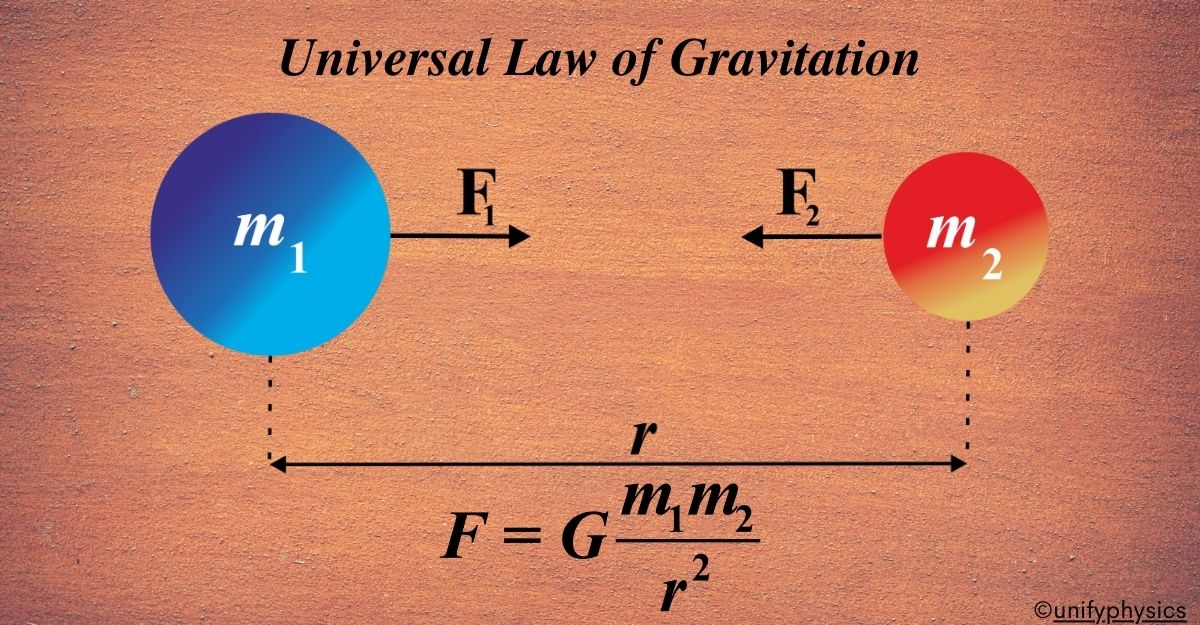
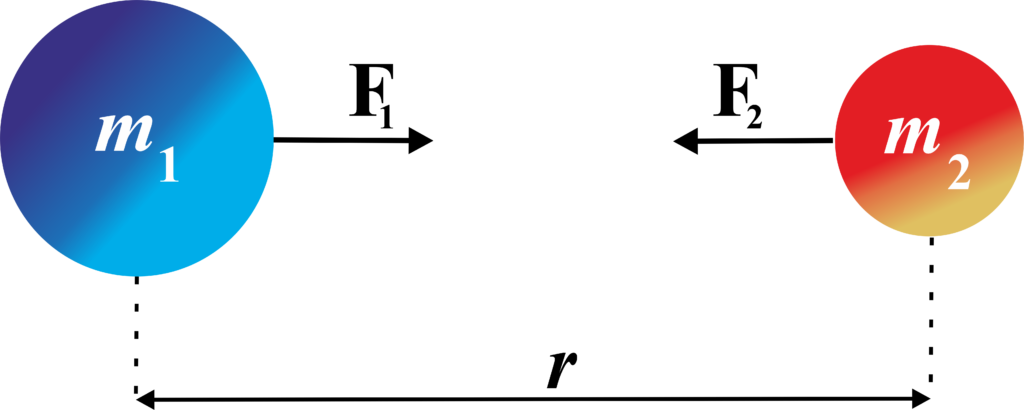
As we delve into the Universal Law of Gravitation, we’ll uncover the mathematical expression that Newton devised to describe this force. This law has had a profound impact on physics and astronomy, paving the way for a deeper understanding of the cosmos and influencing scientific thought for centuries.
Statement
” Every point mass in the universe attracts every other point mass with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between their centers. The force acts along the line joining the two masses.”
Formula
The mathematical expression for the gravitational force (F) between two masses (m1 and m2) separated by a distance (r) is given by:
\(\displaystyle F=G.\frac{{{{m}_{1}}.{{m}_{2}}}}{{{{r}^{2}}}}\)
Where:
- F is the gravitational force,
- G is the gravitational constant (6.674×10−11 Nm2/kg2),
- m1 and m2 are the masses of the two objects,
- r is the distance between their centers.
The Universal Law of Gravitation provides a quantitative description of the force of attraction between any two objects with mass. The force of gravity is directly proportional to the product of the masses and inversely proportional to the square of the distance between their centers. This means that larger masses experience a stronger gravitational force, and the force weakens rapidly as objects move farther apart. Gravity is an attractive force, always acting along the line joining the centers of the two masses. This means that objects with mass are drawn toward each other.
- Falling Objects: The Universal Law of Gravitation explains why objects fall to the ground when released. The Earth’s gravitational pull pulls objects toward its center.
- Planetary Orbits: This law is crucial for understanding the orbits of planets around the Sun. The gravitational force between the Sun and a planet keeps the planet in orbit.
Gravitational force is the driving factor behind the motion of celestial bodies. It governs the orbits of planets around the Sun, the moons around planets, and the interactions between galaxies. Sir Isaac Newton’s formulation of the Universal Law of Gravitation revolutionized our understanding of these celestial dynamics.
Example
Imagine you’re sitting under an apple tree, and you see a ripe apple let go of its branch and start falling to the ground. This everyday occurrence might seem simple, but it’s actually a perfect example of how gravity works, as described by the Universal Law of Gravitation.
In this scenario, we have two main players: the Earth and the apple. Earth, being much larger, has a more powerful gravitational pull. The apple, even though it’s smaller, still has mass, and that means gravity is at play for it too.
Masses Involved:
- Mass of the Earth (mEarth),
- Mass of the apple (mapple).
The distance from the center of the Earth to the center of the apple (r). The gravitational force (F) pulling the apple toward the Earth is given by Newton’s law.
The distance between the center of the Earth and the center of the apple is the key parameter influencing the strength of the gravitational force. As the apple falls, this distance decreases, intensifying the gravitational attraction between the two masses. Newton’s law of gravitation comes into play, expressing the force (F) pulling the apple toward the Earth. The formula,
\(\displaystyle F=G.\frac{{{{m}_{{Earth}}}.{{m}_{{apple}}}}}{{{{r}^{2}}}}\)
outlines the intricate relationship between the masses, the gravitational constant (G), and the square of the distance between their centers. While the mass of the apple is considerably smaller than the Earth, the force exerted by the Earth’s gravity significantly influences the apple’s acceleration. The gravitational force acts as an invisible hand, guiding the apple downward, and the acceleration experienced by the apple is a testament to the gravitational pull defined by Newton’s laws.
This example underscores the universality of the law of gravitation, showing that the same principles governing celestial bodies also shape the behavior of everyday objects on Earth. The Universal Law of Gravitation helps us understand why things fall, making it an essential concept for explaining both everyday events and the movements of celestial bodies in the universe.